(New page: Category:2010 Fall ECE 438 Boutin ---- == Quiz Questions Pool for Week 9 == ---- Q1. * Solution. ---- Q2. * Solution. ---- ...) |
|||
| Line 4: | Line 4: | ||
== Quiz Questions Pool for Week 9 == | == Quiz Questions Pool for Week 9 == | ||
---- | ---- | ||
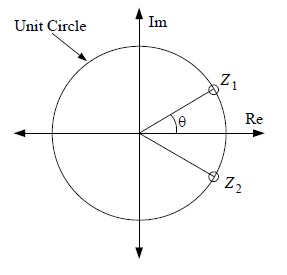
| − | Q1. | + | Q1. Consider the following second order FIR filter with the two zeros on the unit circle as shown below. |
| + | |||
| + | [[Image:Quiz9Q1.jpg]] | ||
| + | |||
| + | The transfer function for this filter is given by <math> H(z) = (1-e^{j\theta}z^{-1})(1-e^{-j\theta}z^{-1})=1-2\cos(\theta)z^{-1}+z^{-2}</math> | ||
| + | |||
| + | :a. Find the difference equation of this filter. | ||
| + | |||
| + | :b. Find the frequency response <math>H(w)</math> by the following two approaches: | ||
| + | ::i. apply the input <math>e^{jwn}</math> to the difference equation describing the system, | ||
| + | ::ii. find the DTFT of the impulse response, | ||
| + | : and verify that both methods lead to the same result. | ||
| + | |||
| + | :c. Find the response of this system to the input | ||
| + | ::<math> | ||
| + | x[n]=\left\{ | ||
| + | \begin{array}{rl} | ||
| + | 1, & \text{ if }n=-2,\\ | ||
| + | 1, & \text{ if }n=0,\\ | ||
| + | 1, & \text{ if }n=2,\\ | ||
| + | 0, & \text{ else. } | ||
| + | \end{array} | ||
| + | \right. | ||
| + | </math> | ||
| + | |||
| + | :d. Is this filter a lowpass, highpass, bandpass or a bandstop filter? | ||
| + | |||
| + | :e. An interference signal modulated at 2kHz and sampled at 8kHz is being inputted to this system and you want to eliminate this interference. What must be the value of <math>\theta</math> to eliminate this signal? | ||
* [[ECE438_Week9_Quiz_Q1sol|Solution]]. | * [[ECE438_Week9_Quiz_Q1sol|Solution]]. | ||
| Line 11: | Line 38: | ||
* [[ECE438_Week9_Quiz_Q2sol|Solution]]. | * [[ECE438_Week9_Quiz_Q2sol|Solution]]. | ||
| + | ---- | ||
| + | Q3. | ||
| + | |||
| + | * [[ECE438_Week9_Quiz_Q3sol|Solution]]. | ||
| + | ---- | ||
| + | Q4. | ||
| + | |||
| + | * [[ECE438_Week9_Quiz_Q4sol|Solution]]. | ||
| + | ---- | ||
| + | Q5. | ||
| + | |||
| + | * [[ECE438_Week9_Quiz_Q5sol|Solution]]. | ||
---- | ---- | ||
Revision as of 10:03, 19 October 2010
Quiz Questions Pool for Week 9
Q1. Consider the following second order FIR filter with the two zeros on the unit circle as shown below.
The transfer function for this filter is given by $ H(z) = (1-e^{j\theta}z^{-1})(1-e^{-j\theta}z^{-1})=1-2\cos(\theta)z^{-1}+z^{-2} $
- a. Find the difference equation of this filter.
- b. Find the frequency response $ H(w) $ by the following two approaches:
- i. apply the input $ e^{jwn} $ to the difference equation describing the system,
- ii. find the DTFT of the impulse response,
- and verify that both methods lead to the same result.
- c. Find the response of this system to the input
- $ x[n]=\left\{ \begin{array}{rl} 1, & \text{ if }n=-2,\\ 1, & \text{ if }n=0,\\ 1, & \text{ if }n=2,\\ 0, & \text{ else. } \end{array} \right. $
- d. Is this filter a lowpass, highpass, bandpass or a bandstop filter?
- e. An interference signal modulated at 2kHz and sampled at 8kHz is being inputted to this system and you want to eliminate this interference. What must be the value of $ \theta $ to eliminate this signal?
Q2.
Q3.
Q4.
Q5.
Back to ECE 438 Fall 2010 Lab Wiki Page
Back to ECE 438 Fall 2010