Rep Function:
A rep function periodically repeats another function with some specified period T ( basically sampling time). Mathematically a rep operator is a function x(t) convoluted with a summation of shifted deltas:
$ rep_T $ = $ x(t)* P_T (t) $
= $ x(t)* \sum_{k=-\infty}^{\infty}\delta(t-kT) $
= $ \sum_{k=-\infty}^{\infty}x(t) * \delta(t-kT) $
= $ \sum_{k=-\infty}^{\infty}x(t-kT) $
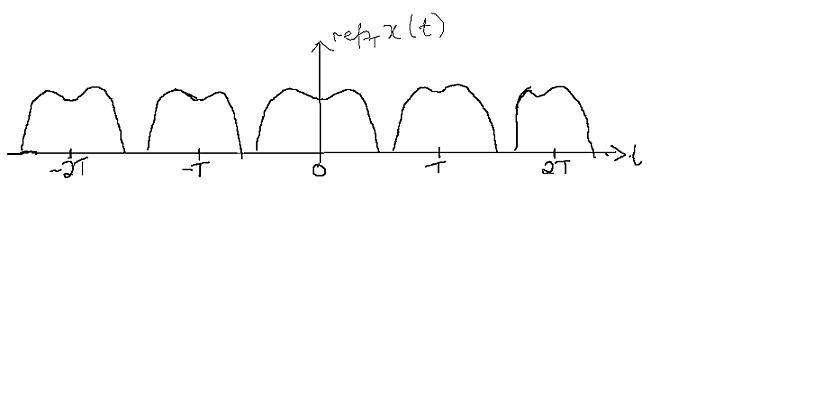
If below is the x(t):
Then the $ rep_T $x(t) looks like:
The resulting function is periodic with period T. To keep x(t) repeating without aliasing, the minimum sampling period should be $ \geqq 2 * T $
Comb Function
A comb function multiplies a function with a periodic train of impulses.
$ comb_T ${x(t)} = $ x(t) . \sum_{k=-\infty}^{\infty}\delta(t-kT) $ = $ x(t) . P_T (t) $