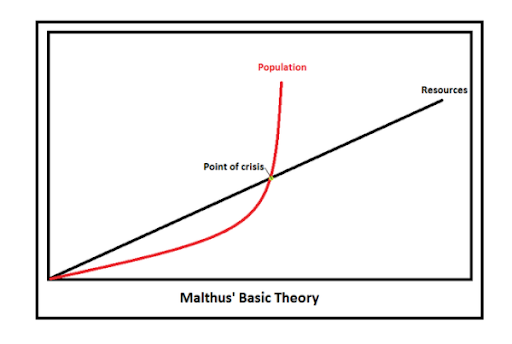
Population Growth:
Malthus’ Theory of Population has limited applicability to modern society. The theory has been criticized and deconstructed in several ways. One such aspect is population growth is not purely exponential. Adolphe Quetelet, a statistician, noted in 1835 that population cannot grow exponentially for a long time (Bacaër 35). Later, in 1838, Verhulst published his Note on the law of population growth. In that paper, Verhulst pointed out that Malthus Theory ignores the increasing difficulty of maintaining a large population. Verhulst claimed that as population becomes larger, growth slows down due to resource limitations. The environment has a finite amount of resources, and the amount of resource determines the maximum population the environment can support. This upper limit is often referred to as “carrying capacity.” When population size is relatively low, it can grow exponentially, in accordance with the Malthus Doctrine. However, as population approaches the environment’s carrying capacity, growth tends to slow down. The population might stabilize at a fixed point near the carrying capacity, or it might go above the capacity. In the second case, positive checks will cause population to decline until it falls below the carrying capacity.
There is significant evidence to support Verhulst’s claims about population growth. According to world bank's data, world’s population over the last 57 years showed linear growth with R2=0.9986. This supports the idea that population growth is not entirely exponential. Verhulst, a French mathematician, checked population census data in France, Belgium, Russia, Essex, and England in the early 19th century and proposed the following equation for population growth to accommodate concerns addressed above (Seidl 398):
$ \frac{dN}{dt}=rN(\frac{K-N}{K}) $
where Nis the population size, Kis the carrying capacity, and ris a growth rate. The growth rate is a parameter that is likely to differ from one population group to another. This equation captures all points stated before. When Nis small relative to K, K-NKis nearly 1, which means growth rate is about rN. As Ngrows towards K, K-NKapproaches 0. When N=K, K-NK=0, and population growth is completely halted. If Ngrows above K, K-NKwould be negative meaning the population would be declining. This makes sense because if the population is greater than the carrying capacity, there would not be enough resources to sustain such a population, and it would therefore decline. The greater Nis the faster the decline. Verhulst’s equation is called the logistic equation, or logistic growth model. It is a better population growth model than Malthus’s purely exponential model.