Slecture rep comb ECE438 Xiaozhe - Rhea

     1.1 Definition of Rep operator
      
         1.1.1 Introduction to Rep operator

         1.1.2 Definition of Rep operator

         1.1.3 Two representations of Rep operator

         1.1.4 Relationship between two representation

     1.2 Definition of Comb operator

         1.1.1 Introduction
     
         1.1.2 Definition of Comb operator

         1.1.3 Two representations of Comb operator

         1.1.4 Relationship between two representation

1.1.1 Introduction to Rep operator

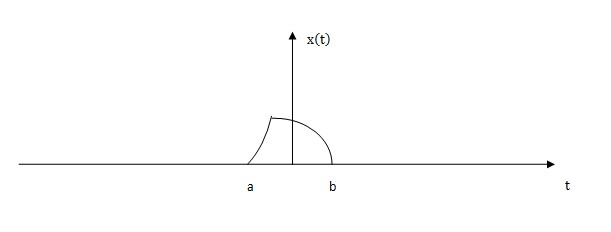
In order to understand Rep operator clearly, a graphical method is introduced as follows:

In the figure above, there is a little pulse with compact supports over a definite interval. When repeating it at capital T, We can get the following figure.

This process which is denoted by Rep operator have the same meaning as taking original function x(t) and shifting it by KT in which K∈N and -∞<K<+∞.

1.1.2 Definition of Rep operator

Rep operator denotes a kind of process which can periodically replicate a function with some specific period T. Where the function has a finite domain of argument and the minimum repeating period T has to > a+b(a denotes left boundary of the graph and b denotes right boundary of the graph ).

1.1.3 Two representations of Rep operator

$ 1.rep_{T}(x[t])=\sum_{k=-\infty}^{\infty}x(t-KT) $

$ 2.rep_{T}(x[t])=x(t) \ast P_{T}(T) $

1.1.4 Relationship between two representation

$ rep_{T}(x[t])=x(t) \ast P_{T}(T) \qquad \qquad \qquad Where \quad P_{T}(T)=\sum_{k=-\infty}^{\infty}\delta(t-KT) $

            $ =x(t) \ast \sum_{k=-\infty}^{\infty}\delta(t-KT) $
            $ =\int_{-\infty}^{\infty}x(\tau)\sum_{k=-\infty}^{\infty}\delta(t-KT-\tau)d\tau $
            $ =\sum_{k=-\infty}^{\infty}\int_{-\infty}^{\infty}x(\tau)\delta(t-KT-\tau)d\tau $

$ Since \quad x(t)=\int_{-\infty}^{\infty}\delta(t-\tau)d\tau $

$ So \quad rep_{T}(x[t])=\sum_{k=-\infty}^{\infty}x(t-KT) $

Post your slecture material here. Guidelines:

• If you wish to post your slecture anonymously, please contact your instructor to get an anonymous login. Otherwise, you will be identifiable through your Purdue CAREER account, and thus you will NOT be anonymous.
• Rephrase the material in your own way, in your own words, based on Prof. Boutin's lecture material.
• Feel free to add your own examples or your own material.
• Focus on the clarity of your explanation. It must be clear, easily understandable.
• Type text using wikitext markup language. Do not post a pdf. Do not upload a word file.
• Type all equations using latex code between <math> </math> tags.
• You may include graphs, pictures, animated graphics, etc.
• You may include links to other Project Rhea pages.

IMPORTANT: DO NOT PLAGIARIZE. If you use other material than Prof. Boutin's lecture material, you must cite your sources. Do not copy text word for word from another source; rephrase everything using your own words. Similarly for graphs, illustrations, pictures, etc. Make your own! Do not copy them from other sources.

(create a question page and put a link below)

Questions and comments

If you have any questions, comments, etc. please post them on this page.