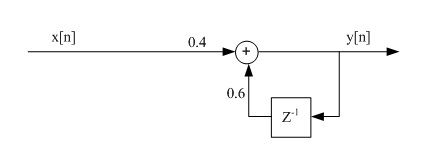
Solution to Q1 of Week 8 Quiz Pool
$ \begin{align} \text{(a)} \quad & y[n] = 0.6 y[n-1] + 0.4 x[n] \\ & h[n] = 0.6h[n-1] + 0.4\delta[n] \\ \end{align}\,\! $
assume that $ h[n]=0 $ when $ n<0 $.
$ \begin{align} {\color{White}abcde} & h[0]=0.2 \\ & h[1]=0.8h[0]=0.2 \times 0.8 \\ & h[2]=0.8h[1]=0.2 \times (0.8)^2 \\ & \ldots \\ & h[n] = 0.2(0.8)^n u[n] \\ \end{align} $
$ \begin{align} \text{(b)} \quad & y[n] = y[n-1] + 0.25 (x[n]-x[n-3]) \\ & h[n] = h[n-1] + 0.25(\delta[n]-\delta[n-3]) \\ \end{align}\,\! $
assume that $ h[n]=0 $ when $ n<0 $.
$ \begin{align} {\color{White}abcde} & h[0]=0.25 \\ & h[1]=h[0]=0.25 \\ & h[2]=h[1]=0.25 \\ & h[3]=h[2]-0.25=0 \\ & h[4]=h[3]=0 \\ & \ldots \\ & h[n] = 0.25(u[n]-u[n-3]) \\ \end{align} $
Credit: Prof. Charles Bouman
Back to Lab Week 8 Quiz Pool
Back to ECE 438 Fall 2010 Lab Wiki Page
Back to ECE 438 Fall 2010