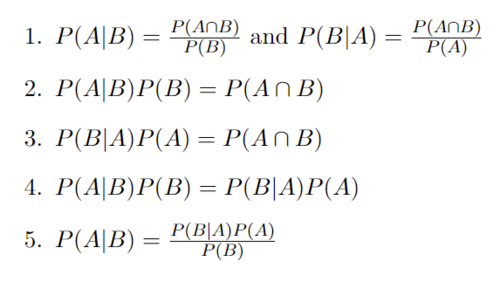| Line 12: | Line 12: | ||
Probability, Conditional Probability, Bayes Theorem | Probability, Conditional Probability, Bayes Theorem | ||
Disease example | Disease example | ||
| + | |||
| + | Definition: Probability is a measure of the likelihood of an event to occur. Many events cannot be predicted with total certainty. We can predict only the chance of an event to occur i.e., how likely they are going to happen, using it. Probability can range from 0 to 1, where 0 means the event to be an impossible one and 1 indicates a certain event. The probability of all the events in a sample space adds up to 1. | ||
Revision as of 10:40, 3 November 2022
Probability : Group 4
Kalpit Patel Rick Jiang Bowen Wang Kyle Arrowood
This is an edit
Probability, Conditional Probability, Bayes Theorem Disease example
Definition: Probability is a measure of the likelihood of an event to occur. Many events cannot be predicted with total certainty. We can predict only the chance of an event to occur i.e., how likely they are going to happen, using it. Probability can range from 0 to 1, where 0 means the event to be an impossible one and 1 indicates a certain event. The probability of all the events in a sample space adds up to 1.
Baye's Theorem Derivation: Starting with the definition of Conditional Probability
Looking at step 1 with the definition of conditional probabilities, it is assumed that P(A) and P(B) are not 0.