| Line 3: | Line 3: | ||
<center> <math> \frac{a+b}{a} = \frac{a}{b} </math> </center> | <center> <math> \frac{a+b}{a} = \frac{a}{b} </math> </center> | ||
| + | |||
| + | That is, the ratio between the sum of two components and the larger part and the ratio between the larger component and smaller component is the same. | ||
After solving for the ratio itself (discussed in the calculations section), we can find that the golden ratio is: | After solving for the ratio itself (discussed in the calculations section), we can find that the golden ratio is: | ||
| − | <center> <math> \frac{1 \pm \sqrt{5}}{2} = \phi = 1. | + | <center> <math> \frac{1 \pm \sqrt{5}}{2} = \phi = 1.61803398875 </math> </center> |
The golden ratio, <math>\phi</math>, is sometimes also called the golden mean or the golden section. The golden ratio can be frequently observed in man-made objects, though they are generally “imperfectly golden” – that is, the ratio is approximately the golden ratio, but not exactly. Some everyday examples include: credit cards, <math>\frac{w}{h}=1.604</math>, and laptop screens, <math>\frac{w}{h}=1.602</math> (Tannenbaum 392). | The golden ratio, <math>\phi</math>, is sometimes also called the golden mean or the golden section. The golden ratio can be frequently observed in man-made objects, though they are generally “imperfectly golden” – that is, the ratio is approximately the golden ratio, but not exactly. Some everyday examples include: credit cards, <math>\frac{w}{h}=1.604</math>, and laptop screens, <math>\frac{w}{h}=1.602</math> (Tannenbaum 392). | ||
Revision as of 16:48, 2 December 2018
Introduction
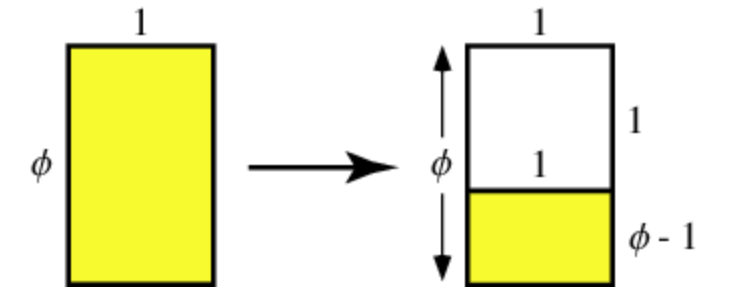
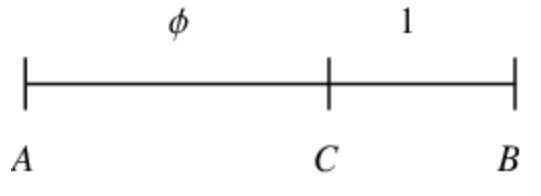
The golden ratio is a ratio such that, given two quantities a and b,
That is, the ratio between the sum of two components and the larger part and the ratio between the larger component and smaller component is the same.
After solving for the ratio itself (discussed in the calculations section), we can find that the golden ratio is:
The golden ratio, $ \phi $, is sometimes also called the golden mean or the golden section. The golden ratio can be frequently observed in man-made objects, though they are generally “imperfectly golden” – that is, the ratio is approximately the golden ratio, but not exactly. Some everyday examples include: credit cards, $ \frac{w}{h}=1.604 $, and laptop screens, $ \frac{w}{h}=1.602 $ (Tannenbaum 392).
Visualizations of the golden ratio can be seen below (Weisstein):
MathIsFun also has an interactive display that can construct a rectangle in the golden ratio given a certain fixed width or length.