| Line 28: | Line 28: | ||
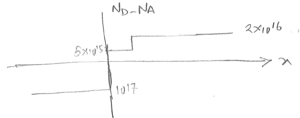
b) <math>x_n<1\mu m</math> | b) <math>x_n<1\mu m</math> | ||
| − | + | ||
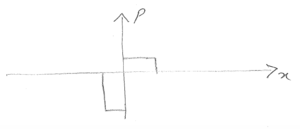
| + | <math>\rho=q(p-n+N_D-N_A)</math> | ||
| + | |||
[[Image:MN2_2012_2.png|Alt text|300x300px]] | [[Image:MN2_2012_2.png|Alt text|300x300px]] | ||
| + | |||
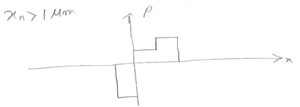
<math>x_n>1\mu m</math> | <math>x_n>1\mu m</math> | ||
| + | |||
[[Image:MN2_2012_3.png|Alt text|300x300px]] | [[Image:MN2_2012_3.png|Alt text|300x300px]] | ||
Latest revision as of 09:52, 6 August 2017
MICROELECTRONICS and NANOTECHNOLOGY (MN)
Question 2: Junction Devices
August 2012
Questions
All questions are in this link
Solutions of all questions
b) $ x_n<1\mu m $
$ \rho=q(p-n+N_D-N_A) $
$ x_n>1\mu m $
c) $ \begin{align*} N_Ax_p&=N_{D1}\cdot1\mu m+N_{D2}(x_n-1)\\ x_n&=1.25\mu m\text{ (chk)} \end{align*} $
d)$ \begin{align*} E_{max}&=\frac{qN_Ax_p}{k_s\epsilon_0}\\ \text{where;}\\ x_p&=\sqrt{\frac{2k_s\epsilon_0}{q}\cdot \frac{N_{D1}}{N_A(N_{D1}+N_A)}\cdot V_{bi}}\\ \text{and }V_{bi}&=\frac{kT}{q}\ln\frac{N_AN_{D1}}{n_i^2} \end{align*} $
e)$ \rho=\begin{cases} 0 &x<-x_p\\ -qN_A &-x_p\le x\le \text{ region 1}\\ qN_{D1} & 0\le x\le x_{n1}\text{ region 2}\\ qN_{D2} & x_{n1}\le x\le x_{n2}\text{ region 3}\\ 0 & x>x_{n2} \end{cases} $ need to solve; $ \frac{dE}{dx}=\frac{\rho}{\epsilon} $
- region 1
$ \int_{E(-x_p)}^{E(x)}dE = \frac{-qN_A}{k_s\epsilon_0}\int_{-x_p}^xdx $ $ \implies E(x)=\frac{-qN_A}{k_s\epsilon_0}(x+x_p) $ at $ x=0 $ $ E(0) = \frac{-qN_A}{k_s\epsilon_0}x_p $
- region 2
$ \int_{E(0)}^{E(x)}dE = \frac{qN_{D1}}{k_s\epsilon_0}\int_{0}^xdx $ $ \implies E(x)=\frac{qN_{D1}}{k_s\epsilon_0}x+\frac{qN_A}{k_s\epsilon_0}x_p $
- region 3
$ \int_{E(x)}^{E(x_{n2})}dE = \frac{qN_{D2}}{k_s\epsilon_0}\int_{x}^{x_{n2}}dx $ $ \implies 0-E(x)=\frac{qN_{D2}}{k_s\epsilon_0}(x_{n2}-x) $ $ \implies E(x)=\frac{qN_{D2}}{k_s\epsilon_0}(x-x_{n2}) $
------------------------------------------------------------------------------------