(Created page with "Category:ECE Category:QE Category:MN Category:problem solving Category:Dynamics <center> <font size= 4> ECE_PhD_Qualifying_Exams|ECE Ph.D. Qualifying Ex...") |
|||
| Line 16: | Line 16: | ||
</font size> | </font size> | ||
| − | August | + | August 2011 |
</center> | </center> | ||
---- | ---- | ||
---- | ---- | ||
==Questions== | ==Questions== | ||
| − | All questions are in this [https://engineering.purdue.edu/ECE/Academics/Graduates/ | + | All questions are in this [https://engineering.purdue.edu/ECE/Academics/Graduates/Archived_QE_August_11/MN-2%20QE%2011.pdf link] |
=Solutions of all questions= | =Solutions of all questions= | ||
| − | 1) | + | 1) a) Direct bandgap semiconductors used in Lasers and LEDs. |
| − | + | Photodetectors. | |
| − | + | b) Si has a lattice matched <math>SiO_2</math> to reduce surface defects. | |
| + | Si is almost 99\% pure. | ||
| − | + | c) <math>\sim 9eV</math> | |
| − | + | d) (2\:\:\:\:1\:\:\:\:0) | |
| − | <math>\ | + | <math>\rightarrow \:\frac{1}{2}\:\:\:\:1\:\:\:\:\infty </math> |
| − | + | <math>\rightarrow \:1\:\:\:\:2\:\:\:\:\infty</math> | |
| + | [[Image:MN2_2011_1.png|Alt text|300x300px]] | ||
| − | + | e) | |
| − | \ | + | <math>\sim 900-1200</math> deg C. |
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | </math> | + | |
------------------------------------------------------------------------------------ | ------------------------------------------------------------------------------------ | ||
2) | 2) | ||
| + | Neutral p | ||
<math> | <math> | ||
\begin{align*} | \begin{align*} | ||
| − | + | p_0&=10^{15}\\ | |
| − | + | n_0&=\frac{10^{20}}{10^{15}}=10^5 | |
| − | + | \end{align*} | |
| − | & | + | </math> |
| + | Neutral n | ||
| + | <math> | ||
| + | \begin{align*} | ||
| + | n_0&=10^{18}\\ | ||
| + | p_0&=\frac{10^{20}}{10^{18}}=10^2 | ||
\end{align*} | \end{align*} | ||
</math> | </math> | ||
| Line 60: | Line 61: | ||
------------------------------------------------------------------------------------ | ------------------------------------------------------------------------------------ | ||
3) | 3) | ||
| − | |||
<math> | <math> | ||
| − | \ | + | \begin{align*} |
| + | x_{n0}\cdot 10^{18}&=x_{p0}\cdot10^{15}\\ | ||
| + | \implies x_{p0}&=10^3\times0.001\mu m = 1\mu m | ||
| + | \end{align*} | ||
</math> | </math> | ||
| − | + | ------------------------------------------------------------------------------------ | |
| − | + | 4) <math> | |
| − | + | x_n = \sqrt{\frac{2\epsilon}{q}\frac{N_A}{N_D(N_A+N_D)}\cdot(V_{bi}+V)} | |
| − | < | + | |
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
</math> | </math> | ||
------------------------------------------------------------------------------------ | ------------------------------------------------------------------------------------ | ||
| − | + | 5) | |
| + | <math> | ||
| + | \begin{align*} | ||
| + | \rho &=\frac{1}{q\mu_nN_D} = \frac{1}{2\times10^{-19}\times500\times10^{18}}\\ | ||
| + | &=\frac{1}{1000\times10^{-1}}\Omega\cdot cm\\ | ||
| + | &=10^{-2}\Omega\cdot cm | ||
| + | \end{align*} | ||
| + | </math> | ||
| + | <math> | ||
| + | R_s=\frac{\rho}{L} =\frac{10^{-2}}{5\times10^{-4}} = 20\Omega/\Box | ||
| + | </math> | ||
| + | |||
| + | ------------------------------------------------------------------------------------ | ||
| + | 6) | ||
<math> | <math> | ||
| − | \ | + | \begin{align*} |
| + | J_n&=q_n\mu_nE\\ | ||
| + | &=2\times10^{-19}\times10^{18}\times500\times1000\\ | ||
| + | &=10^5 A/cm^2 | ||
| + | \end{align*} | ||
</math> | </math> | ||
| + | |||
| + | ------------------------------------------------------------------------------------ | ||
| + | 7) | ||
| + | <math> | ||
| + | \begin{align*} | ||
| + | J_{diff} &=qD_n\frac{d\triangle n}{dx}\\ | ||
| + | &=2\times10^{-19}\times500\times 25m\times\frac{\triangle n_p}{5\mu m}\\ | ||
| + | \end{align*} | ||
| + | </math> | ||
| + | |||
| + | ------------------------------------------------------------------------------------ | ||
| + | 8)[[Image:MN2_2011_2.png|Alt text|300x300px]] | ||
| + | ------------------------------------------------------------------------------------ | ||
| + | 9)[[Image:MN2_2011_3.png|Alt text|300x300px]] | ||
| + | ------------------------------------------------------------------------------------ | ||
| + | 10) Forward Active Mode | ||
| + | [[Image:MN2_2011_4.png|Alt text|300x300px]] | ||
| + | ------------------------------------------------------------------------------------ | ||
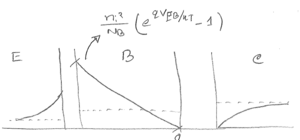
| + | 11)Fig 11.15(SDF) Page 425 | ||
| + | [[Image:MN2_2011_5.png|Alt text|300x300px]] | ||
| + | <math>\beta </math> is reduced by both phenomena. | ||
------------------------------------------------------------------------------------ | ------------------------------------------------------------------------------------ | ||
| + | 12)[[Image:MN2_2011_6.png|Alt text|300x300px]] | ||
| + | |||
---- | ---- | ||
[[ECE_PhD_Qualifying_Exams|Back to ECE Qualifying Exams (QE) page]] | [[ECE_PhD_Qualifying_Exams|Back to ECE Qualifying Exams (QE) page]] | ||
Revision as of 21:20, 5 August 2017
MICROELECTRONICS and NANOTECHNOLOGY (MN)
Question 2: Junction Devices
August 2011
Questions
All questions are in this link
Solutions of all questions
1) a) Direct bandgap semiconductors used in Lasers and LEDs. Photodetectors.
b) Si has a lattice matched $ SiO_2 $ to reduce surface defects. Si is almost 99\% pure.
c) $ \sim 9eV $
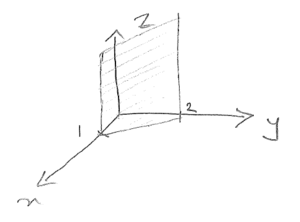
d) (2\:\:\:\:1\:\:\:\:0) $ \rightarrow \:\frac{1}{2}\:\:\:\:1\:\:\:\:\infty $
$ \rightarrow \:1\:\:\:\:2\:\:\:\:\infty $
e) $ \sim 900-1200 $ deg C.
------------------------------------------------------------------------------------
2) Neutral p $ \begin{align*} p_0&=10^{15}\\ n_0&=\frac{10^{20}}{10^{15}}=10^5 \end{align*} $ Neutral n $ \begin{align*} n_0&=10^{18}\\ p_0&=\frac{10^{20}}{10^{18}}=10^2 \end{align*} $
------------------------------------------------------------------------------------
3)
$ \begin{align*} x_{n0}\cdot 10^{18}&=x_{p0}\cdot10^{15}\\ \implies x_{p0}&=10^3\times0.001\mu m = 1\mu m \end{align*} $
------------------------------------------------------------------------------------
4) $ x_n = \sqrt{\frac{2\epsilon}{q}\frac{N_A}{N_D(N_A+N_D)}\cdot(V_{bi}+V)} $
------------------------------------------------------------------------------------
5) $ \begin{align*} \rho &=\frac{1}{q\mu_nN_D} = \frac{1}{2\times10^{-19}\times500\times10^{18}}\\ &=\frac{1}{1000\times10^{-1}}\Omega\cdot cm\\ &=10^{-2}\Omega\cdot cm \end{align*} $ $ R_s=\frac{\rho}{L} =\frac{10^{-2}}{5\times10^{-4}} = 20\Omega/\Box $
------------------------------------------------------------------------------------ 6) $ \begin{align*} J_n&=q_n\mu_nE\\ &=2\times10^{-19}\times10^{18}\times500\times1000\\ &=10^5 A/cm^2 \end{align*} $ ------------------------------------------------------------------------------------ 7) $ \begin{align*} J_{diff} &=qD_n\frac{d\triangle n}{dx}\\ &=2\times10^{-19}\times500\times 25m\times\frac{\triangle n_p}{5\mu m}\\ \end{align*} $ ------------------------------------------------------------------------------------ 8)------------------------------------------------------------------------------------ 9)
------------------------------------------------------------------------------------ 10) Forward Active Mode
------------------------------------------------------------------------------------ 11)Fig 11.15(SDF) Page 425
$ \beta $ is reduced by both phenomena.
------------------------------------------------------------------------------------ 12)