| Line 16: | Line 16: | ||
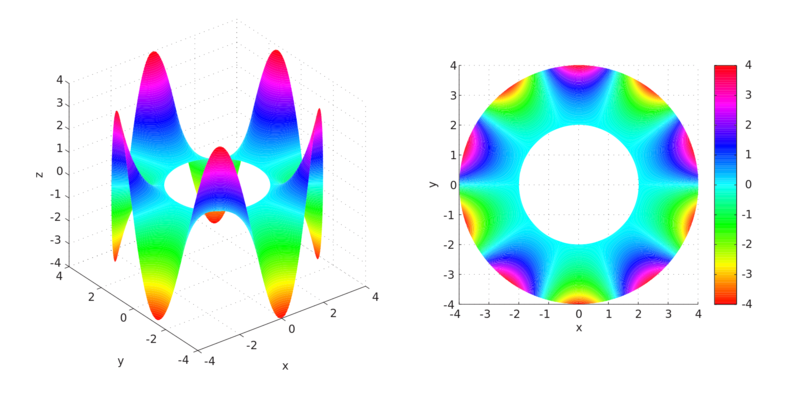
[[File:800px-Laplace's_equation_on_an_annulus.svg.png|center|Example of Harmonic Function]] | [[File:800px-Laplace's_equation_on_an_annulus.svg.png|center|Example of Harmonic Function]] | ||
| + | |||
| + | Harmonic Function in x, y, and z. | ||
</center> | </center> | ||
| + | |||
| + | |||
[[Walther_MA271_Fall2020_topic9|Back to main page]] | [[Walther_MA271_Fall2020_topic9|Back to main page]] | ||
Revision as of 18:05, 6 December 2020
Applications: Harmonic Functions
Definition
Harmonic functions are functions that satisfy the equation
$ \frac{\partial^{2} f}{\partial x_{1}^{2}}+\frac{\partial^{2} f}{\partial x_{2}^{2}}+\cdots+\frac{\partial^{2} f}{\partial x_{n}^{2}}=0 $, or $ \large\Delta f = \nabla^{2} f = 0 $.
A concurrent definition for a harmonic function is the idea that the value at a point in a function is always equal to the average of the values along a circle surrounding that point. This leads to an interesting conclusion about the Laplace Operator itself, in that when $ \Delta f = 0 $, the above statement is true.
Harmonic Function in x, y, and z.