| Line 9: | Line 9: | ||
The golden angle is simply the golden ratio applied to a circle: it is the smaller angle formed by two arcs that are related by the golden ratio. | The golden angle is simply the golden ratio applied to a circle: it is the smaller angle formed by two arcs that are related by the golden ratio. | ||
| − | <center> <math> | + | <center> <math> \frac{a}{b} = \frac{a+b}{a} = \frac{360^o}{\theta} = \frac{1}{\phi} </math> </center> |
<br><br>[[Category:MA279Fall2018Walther]] | <br><br>[[Category:MA279Fall2018Walther]] | ||
Revision as of 19:05, 1 December 2018
The Fibonacci Sequence in Leaves
The Fibonacci sequence is present in both the structure and arrangement of leaves in many plants. Since plants rely on photosynthesis, they want to maximize the amount of sunlight that strikes their leaves. The vertical growth of many plants means that leaves can cover up each other. To minimize this effect, the leaves are grown such that the angle between each successive leaf is the golden angle, as shown in Figure 2.

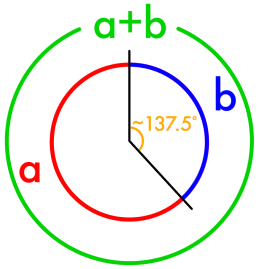
The golden angle is simply the golden ratio applied to a circle: it is the smaller angle formed by two arcs that are related by the golden ratio.

