(Added complete solution) |
m (Fixed appearance of i_1(t) cancellation) |
||
| Line 31: | Line 31: | ||
<math>\begin{align} | <math>\begin{align} | ||
v_1(t) &= r_1 \cancelto{0}{i_1(t)} + \mathit{p}\lambda_1(t) \\ | v_1(t) &= r_1 \cancelto{0}{i_1(t)} + \mathit{p}\lambda_1(t) \\ | ||
| − | v_1(t) &= \frac{d}{dt} \frac{1}{2}N^2 \mu_0 \ell (\cancelto{i_1(t)} + i_2(t)) G(g) \\ | + | v_1(t) &= \frac{d}{dt} \frac{1}{2}N^2 \mu_0 \ell (\cancelto{0}{i_1(t)} + i_2(t)) G(g) \\ |
v_1(t) &= \frac{1}{2}N^2 \mu_0 \ell G(g) \frac{d}{dt} \left[I_2 \cos(\omega_e t)\right] \\ | v_1(t) &= \frac{1}{2}N^2 \mu_0 \ell G(g) \frac{d}{dt} \left[I_2 \cos(\omega_e t)\right] \\ | ||
v_1(t) &= -\frac{1}{2}N^2 \mu_0 \ell G(g) I_2 \omega_e \sin(\omega_e t) | v_1(t) &= -\frac{1}{2}N^2 \mu_0 \ell G(g) I_2 \omega_e \sin(\omega_e t) | ||
Latest revision as of 13:22, 22 January 2018
Answers and Discussions for
Contents
Recycled Information from Problem 1
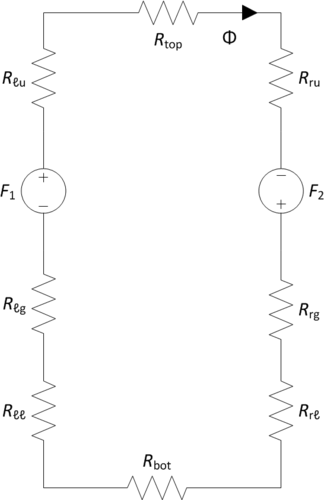
Create and Solve Magnetic Equivalent Circuit
$ \begin{align} G(g) &= \frac{\mu_r w_u d_u}{2 d_s d_u + d_u^2 + w_s w_u + w_u^2 + \mu_r d_u g} \\ \lambda_1(i_1,i_2,g) &= \frac{1}{2}N^2 \mu_0 \ell (i_1 + i_2) G(g) \\ \lambda_2(i_1,i_2,g) &= \lambda_1(i_1,i_2,g) \end{align} $
Problem 2
The magnetic equivalent circuit (MEC) used in Problem 1 provides useful information for Problem 2. The current waveforms are $ i_1(t) = 0 \; \forall t $ (open circuited) and $ i_2(t) = I_2 \cos(\omega_e t) $ while the airgap distance $ g $ remains fixed.
The voltage equation for the first coil may be written down.
$ \begin{align} v_1(t) &= r_1 \cancelto{0}{i_1(t)} + \mathit{p}\lambda_1(t) \\ v_1(t) &= \frac{d}{dt} \frac{1}{2}N^2 \mu_0 \ell (\cancelto{0}{i_1(t)} + i_2(t)) G(g) \\ v_1(t) &= \frac{1}{2}N^2 \mu_0 \ell G(g) \frac{d}{dt} \left[I_2 \cos(\omega_e t)\right] \\ v_1(t) &= -\frac{1}{2}N^2 \mu_0 \ell G(g) I_2 \omega_e \sin(\omega_e t) \end{align} $
The answer to this problem is mostly just substitution and a time derivative of a sinusoid.
$ \begin{equation} \boxed{v_1(t) = -\frac{1}{2} \frac{N^2 \mu_r \mu_0 \ell w_u d_u}{2 d_s d_u + d_u^2 + w_s w_u + w_u^2 + \mu_r d_u g} \omega_e I_2 \sin(\omega_e t)} \end{equation} $