Difference between revisions of "ECE PhD QE ES1 2009 Problem1" - Rhea

Revision as of 21:53, 16 January 2018

Answers and Discussions for

ECE Ph.D. Qualifying Exam ES-1 August 2009

Problem 1

Create and Solve Magnetic Equivalent Circuit

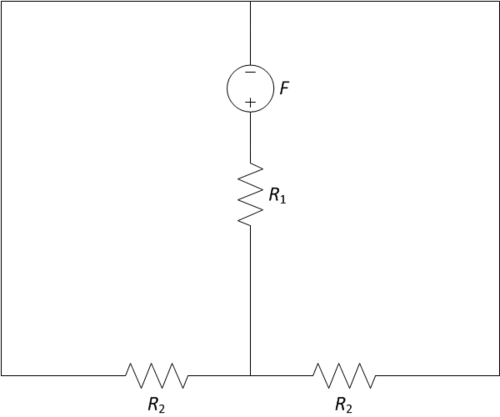
A magnetic equivalent circuit (MEC) needs to be constructed from the given plunger geometry. A magnetomotive force of $\mathcal{F} = Ni$ acts as an analogue of a DC voltage source. In general, a reluctance may be expressed as $\mathcal{R} = \frac{\ell}{\mu A}$ and function as a resistance analogue. If the steel is infinitely permeable, then the reluctance associated with a path through steel is zero (ideal conductor equivalent). In this problem, the permeability of air (linear, isotropic, and homogeneous) is approximated as free space: $\mu_{\text{air}} = \mu_{r,\text{air}} \mu_0 \approx \mu_0$ . Without any fringing or leakage considered, magnetic flux $\Phi$ will more or less follow the direction of the steel.

The reluctances need to be calculated using the length and cross sectional area presented to each path in air.

$\mathcal{R}_1(g) = \frac{g}{\mu_0 w d}$
$\mathcal{R}_2 = \frac{c}{\mu_0 t d}$

The total magnetic flux passing through $\mathcal{F}$ needs to be found. It should be apparent that $\mathcal{R}_1$ is in series with the parallel combination of $\mathcal{R}_2$ and $\mathcal{R}_2$ .

$\begin{align} \Phi_1(i,g) &= \frac{\mathcal{F}}{\mathcal{R}_{eq}} \\ \Phi_1(i,g) &= \frac{Ni}{\mathcal{R}_1 + \frac{1}{2}\mathcal{R}_2} \\ \Phi_1(i,g) &= \frac{Ni}{\frac{g}{\mu_0 w d} + \frac{c}{2\mu_0 t d}} \\ \Phi_1(i,g) &= \frac{Ni}{\frac{2 t g}{2\mu_0 w t d} + \frac{w c}{2\mu_0 w t d}} \\ \Phi_1(i,g) &= \frac{2N \mu_0 w t d i}{2 t g + w c} \end{align}$

The magnetic flux linkage of the coil follows readily.

$\begin{align} \lambda_1(i,g) &= N \Phi_1(i,g) \\ \lambda_1(i,g) &= \frac{2N^2 \mu_0 w t d i}{2 t g + w c} \end{align}$

Coenergy Calculation

Coenergy will be calculated in steps using a calculus of variations process. Before any steps can be completed, the contribution to coenergy of fixing the mechanical system should be documented (zero unless the mechanical system can store energy itself by moving from one position to another).

$\begin{equation} W_{c,0} = 0 \end{equation}$

The first step in the coenergy calculation will ramp dummy variable $$ i_1' $$ from $$ 0 $$ to its final value of $$ i $$ .

$\begin{align} W_{c,1} &= \int_{i_1' = 0}^{i} \lambda_1\left(i_1', g\right) \, di_1' \\ W_{c,1} &= \int_{i_1' = 0}^{i} \frac{2N^2 \mu_0 w t d \cdot i_1'}{2 t g + w c} \, di_1' \\ W_{c,1} &= \frac{N^2 \mu_0 w t d \cdot i^2}{2 t g + w c} - 0 \end{align}$

The final step is the sum all the individual coenergy contributions $W_c(i, g) = \sum_{k = 0}^{1} W_{c,k}(i, g)$ .

$\begin{align} W_c(i, g) &= W_{c,0} + W_{c,1} \\ W_c(i, g) &= 0 + \frac{N^2 \mu_0 w t d \cdot i^2}{2 t g + w c} \\ W_c(i, g) &= \frac{N^2 \mu_0 w t d i^2}{2 t g + w c} \end{align}$

This calculation is consistent with the known result of a magnetically linear system having a coenergy of $W_c(i, g) = \frac{1}{2} L_1(g) i^2 = \frac{1}{2} \lambda_1(i, g) i$ .

Electromechanical Force Calculation

Electromechanical force is just the partial derivative of coenergy with respect to mechanical position, the variable $$ g $$ in this problem.

$\begin{align} f_e &= \frac{\partial W_c(i, g)}{\partial g} \\ f_e &= \frac{N^2 \mu_0 w t d i^2}{\left(2 t g + w c\right)^2} \cdot -2t \end{align}$

Recall by the Quotient Rule or the Chain Rule + Power Rule that $\frac{d}{dx} \frac{a}{bx + c} = \frac{-ab}{(bx + c)^2}$ for $a,b,c \in \mathbb{R}$ . Thus, the electromechanical force equation is obtained for this device.

$\begin{equation} \boxed{T_e = \frac{-2 N^2 \mu_0 w t^2 d i^2}{\left(2 t g + w c\right)^2}} \end{equation}$

Discussion

Back to ES-1, August 2009

@@ Line 41: / Line 41: @@
 ===<small>Coenergy Calculation</small>===
-<!--
-The mechanical rotor position <math>\theta_{rm}</math> is such that <math>\theta_r = \theta_{rm}</math>, implying that the number of poles is <math>P = 2</math>. Coenergy will be calculated in steps using a '''calculus of variations''' process. Before any steps can be completed, the contribution to coenergy of fixing the mechanical system should be documented (zero unless the mechanical system can store energy).
+Coenergy will be calculated in steps using a '''calculus of variations''' process. Before any steps can be completed, the contribution to coenergy of fixing the mechanical system should be documented (zero unless the mechanical system can store energy itself by moving from one position to another).
 <math>\begin{equation}
@@ Line 48: / Line 48: @@
 \end{equation}</math>
-The first step in the coenergy calculation will ramp dummy variable <math>i_1'</math> from <math>0</math> to its final value of <math>i_1</math> while dummy variable <math>i_2' = 0</math> is held at its initial value. Substitute <math>i_m = i_1 + 2i_2</math> as needed.
+The first step in the coenergy calculation will ramp dummy variable <math>i_1'</math> from <math>0</math> to its final value of <math>i</math>.
 <math>\begin{align}
-W_{c,1} &= \left.\int_{i_1' = 0}^{i_1} \lambda_1\left(i_1', i_2', \theta_r\right) \, di_1'\right|_{i_2' = 0} + \left.\int_{i_1' = 0}^{i_1} \lambda_2\left(i_1', i_2', \theta_r\right) \, di_1'\right|_{i_2' = 0} \\
+W_{c,1} &= \int_{i_1' = 0}^{i} \lambda_1\left(i_1', g\right) \, di_1' \\
-W_{c,1} &= \int_{i_1' = 0}^{i_1} \left[5i_1' + 10\left(1 - \frac{1}{1 + i_1' + \cancelto{0}{2i_2'}}\right) \cos(\theta_r)\right] \, di_1' + \int_{i_1' = 0}^{i_1} \left[\cancelto{0}{i_2'} + 20\left(1 - \frac{1}{1 + i_1' + \cancelto{0}{2i_2'}}\right) \cos(\theta_r)\right] \, di_1' \\
+W_{c,1} &= \int_{i_1' = 0}^{i} \frac{2N^2 \mu_0 w t d \cdot i_1'}{2 t g + w c} \, di_1' \\
-W_{c,1} &= \frac{5}{2} i_1^2 + 10\left(i_1 - \ln|1 + i_1| + \cancelto{0}{\ln|1 + 0|}\right) \cos(\theta_r) + 20\left(i_1 - \ln|1 + i_1| + \cancelto{0}{\ln|1 + 0|}\right) \cos(\theta_r) \\
+W_{c,1} &= \frac{N^2 \mu_0 w t d \cdot i^2}{2 t g + w c} - 0
-W_{c,1} &= \frac{5}{2} i_1^2 + 10\left(i_1 - \ln|1 + i_1|\right) \cos(\theta_r) + 20\left(i_1 - \ln|1 + i_1|\right) \cos(\theta_r)
 \end{align}</math>
-The second step in the coenergy calculation will ramp dummy variable <math>i_2'</math> from <math>0</math> to its final value of <math>i_2</math> while dummy variable <math>i_1' = i_1</math> is held at its final value. Substitute <math>i_m = i_1 + 2i_2</math> as needed.
+The final step is the sum all the individual coenergy contributions <math>W_c(i, g) = \sum_{k = 0}^{1} W_{c,k}(i, g)</math>.
 <math>\begin{align}
-W_{c,2} &= \left.\int_{i_2' = 0}^{i_2} \lambda_1\left(i_1', i_2', \theta_r\right) \, di_2'\right|_{i_1' = i_1} + \left.\int_{i_2' = 0}^{i_2} \lambda_2\left(i_1', i_2', \theta_r\right) \, di_2'\right|_{i_1' = i_1} \\
+W_c(i, g) &= W_{c,0} + W_{c,1} \\
-W_{c,2} &= \int_{i_2' = 0}^{i_2} \left[5i_1 + 10\left(1 - \frac{1}{1 + i_1 + 2i_2'}\right) \cos(\theta_r)\right] \, di_2' + \int_{i_2' = 0}^{i_2} \left[i_2' + 20\left(1 - \frac{1}{1 + i_1 + 2i_2'}\right) \cos(\theta_r)\right] \, di_2' \\
+W_c(i, g) &= 0 + \frac{N^2 \mu_0 w t d \cdot i^2}{2 t g + w c} \\
-W_{c,2} &= 5 i_1 i_2 + 10\left(i_2 - \ln|1 + i_1 + 2i_2| + \ln|1 + i_1|\right) \cos(\theta_r) + \frac{1}{2} i_2^2 + 20\left(i_2 - \ln|1 + i_1 + 2i_2| + \ln|1 + i_1|\right) \cos(\theta_r)
+W_c(i, g) &= \frac{N^2 \mu_0 w t d i^2}{2 t g + w c}
 \end{align}</math>
-The final step is the sum all the individual coenergy contributions <math>W_c(i_1, i_2, \theta_r) = \sum_{k = 0}^{2} W_{c,k}(i_1, i_2, \theta_r)</math>. Recall that <math>i_1, i_2 \geq 0</math> to simplify the absolute value signs.
+This calculation is consistent with the known result of a magnetically linear system having a coenergy of <math>W_c(i, g) = \frac{1}{2} L_1(g) i^2 = \frac{1}{2} \lambda_1(i, g) i</math>.
-<math>\begin{align}
+===<small>Electromechanical Force Calculation</small>===
-W_c(i_1, i_2, \theta_r) &= W_{c,0} + W_{c,1} + W_{c,2} \\
-W_c(i_1, i_2, \theta_r) &=
-\begin{split}
-   &{}0 + \frac{5}{2} i_1^2 + 10\left(i_1 - \cancel{\ln|1 + i_1|}\right) \cos(\theta_r) + 20\left(i_1 - \cancel{\ln|1 + i_1|}\right) \cos(\theta_r) \\
-   &{}+ 5 i_1 i_2 + 10\left(i_2 - \ln|1 + i_1 + 2i_2| + \cancel{\ln|1 + i_1|}\right) \cos(\theta_r) \\
-   &{}+ \frac{1}{2} i_2^2 + 20\left(i_2 - \ln|1 + i_1 + 2i_2| + \cancel{\ln|1 + i_1|}\right) \cos(\theta_r)
-\end{split} \\
-W_c(i_1, i_2, \theta_r) &= \frac{5}{2} i_1^2 + 5 i_1 i_2 + \frac{1}{2} i_2^2 + 10\left[i_1 + i_2 - \ln(1 + i_1 + 2i_2)\right] \cos(\theta_r) + 20\left[i_1 + i_2 - \ln(1 + i_1 + 2i_2)\right] \cos(\theta_r)
-\end{align}</math>
-The last two terms have the same variable dependence, so the coefficients may be added.
+Electromechanical force is just the partial derivative of coenergy with respect to mechanical position, the variable <math>g</math> in this problem.
-<math>\begin{equation}
-\boxed{W_c(i_1, i_2, \theta_r) = \frac{5}{2} i_1^2 + 5 i_1 i_2 + \frac{1}{2} i_2^2 + 30\cos(\theta_r)\left[i_1 + i_2 - \ln(1 + i_1 + 2i_2)\right]}
-\end{equation}</math>
-===<small>Electromechanical Torque Calculation</small>===
-Electromechanical torque is just the partial derivative of coenergy with respect to mechanical rotor position.
 <math>\begin{align}
-T_e &= \frac{\partial W_c(i_1, i_2, \theta_r)}{\partial \cancelto{\theta_r}{\theta_{rm}}} \\
+f_e &= \frac{\partial W_c(i, g)}{\partial g} \\
-T_e &= 0 + 0 + 0 - 30\sin(\theta_r)\left[i_1 + i_2 - \ln(1 + i_1 + 2i_2)\right]
+f_e &= \frac{N^2 \mu_0 w t d i^2}{\left(2 t g + w c\right)^2} \cdot -2t
 \end{align}</math>
-Thus, the electromechanical torque equation is obtained for this device.
+Recall by the Quotient Rule or the Chain Rule + Power Rule that <math>\frac{d}{dx} \frac{a}{bx + c} = \frac{-ab}{(bx + c)^2}</math> for <math>a,b,c \in \mathbb{R}</math>. Thus, the electromechanical force equation is obtained for this device.
 <math>\begin{equation}
-\boxed{T_e = -30\sin(\theta_r)\left[i_1 + i_2 - \ln(1 + i_1 + 2i_2)\right]}
+\boxed{T_e = \frac{-2 N^2 \mu_0 w t^2 d i^2}{\left(2 t g + w c\right)^2}}
 \end{equation}</math>
--->
 ----
 ==Discussion==