| Line 6: | Line 6: | ||
a) | a) | ||
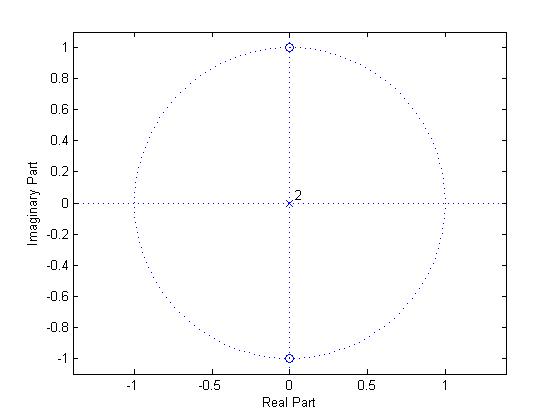
| − | <math>y[n]= \frac{x[n]+x[n-1]}{2} </math | + | <math>y[n]= \frac{x[n]+x[n-1]}{2} </math> |
| − | Applying Z-transform on both sides and grouping terms, we can obtain the transfer function | + | Applying Z-transform on both sides and grouping terms, we can obtain the transfer function |
<math>\begin{align} | <math>\begin{align} | ||
| Line 17: | Line 17: | ||
Frequency Response <math>H(\omega)</math> | Frequency Response <math>H(\omega)</math> | ||
| + | |||
<math>\begin{align} | <math>\begin{align} | ||
H[e^{j\omega }] &= \frac{1+e^{-j\omega }}{2} \\ | H[e^{j\omega }] &= \frac{1+e^{-j\omega }}{2} \\ | ||
| Line 29: | Line 30: | ||
<math>y[n]= \frac{x[n]-x[n-1]}{2}</math> | <math>y[n]= \frac{x[n]-x[n-1]}{2}</math> | ||
| − | Applying Z-transform on both sides and grouping terms, we can obtain the transfer function | + | Applying Z-transform on both sides and grouping terms, we can obtain the transfer function |
<math>\begin{align} | <math>\begin{align} | ||
| Line 38: | Line 39: | ||
Frequency Response <math>H(\omega)</math> | Frequency Response <math>H(\omega)</math> | ||
| + | |||
<math>\begin{align} | <math>\begin{align} | ||
H[e^{j\omega }] &= \frac{1-e^{-j\omega }}{2} \\ | H[e^{j\omega }] &= \frac{1-e^{-j\omega }}{2} \\ | ||
Revision as of 10:15, 29 October 2011
Contents
Homework 6, ECE438, Fall 2011, Prof. Boutin
Question 1
a)
$ y[n]= \frac{x[n]+x[n-1]}{2} $
Applying Z-transform on both sides and grouping terms, we can obtain the transfer function
$ \begin{align} Y[z]&= \frac{X[z]+X[z].z^{-1}}{2} \\ \frac{Y[z]}{X[z]}&= \frac{1+z^{-1}}{2} \\ H[z] &= \frac{1+z^{-1}}{2} \\ \end{align} $
Frequency Response $ H(\omega) $
$ \begin{align} H[e^{j\omega }] &= \frac{1+e^{-j\omega }}{2} \\ &= e^{-j\frac{\omega }{2}} \left( \frac{e^{j\frac{\omega }{2}}+e^{-j\frac{\omega }{2}}}{2} \right) \\ &= e^{-j\frac{\omega }{2}} cos \left( \frac{\omega }{2} \right) \\ \end{align} $
b)
$ y[n]= \frac{x[n]-x[n-1]}{2} $
Applying Z-transform on both sides and grouping terms, we can obtain the transfer function
$ \begin{align} Y[z]&= \frac{X[z]-X[z].z^{-1}}{2} \\ \frac{Y_2[z]}{X[z]}&= \frac{1-z^{-1}}{2} \\ H[z] &= \frac{1-z^{-1}}{2} \\ \end{align} $
Frequency Response $ H(\omega) $
$ \begin{align} H[e^{j\omega }] &= \frac{1-e^{-j\omega }}{2} \\ &= e^{-j\frac{\omega }{2}} \left( \frac{e^{j\frac{\omega }{2}}-e^{-j\frac{\omega }{2}}}{2} \right) \\ &= je^{-j\frac{\omega }{2}} \left( \frac{e^{j\frac{\omega }{2}}-e^{-j\frac{\omega }{2}}}{2j} \right) \\ &= je^{-j\frac{\omega }{2}} sin \left( \frac{\omega }{2} \right) \\ \end{align} $
Question 2
Question 3
Question 4
Question 5
Back to Homework 6
Back to ECE 438 Fall 2011