(→Part A: Periodic Signals Revisited) |
(→Creating a Periodic from a Non-Periodic Function) |
||
| (4 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
== Part A: Periodic Signals Revisited == | == Part A: Periodic Signals Revisited == | ||
| + | === Periodic Continuous Time Signal === | ||
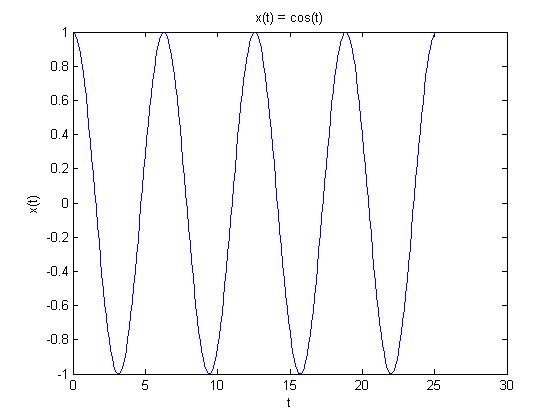
I used the continuous time signal <math> x(t) = cos(t) </math>, as it seemed many people used in Homework 1 for their example of a periodic function. The signal repeats itself at intervals of <math> 2\pi </math>. | I used the continuous time signal <math> x(t) = cos(t) </math>, as it seemed many people used in Homework 1 for their example of a periodic function. The signal repeats itself at intervals of <math> 2\pi </math>. | ||
[[Image:HW2_CTfunction_ECE301Fall2008mboutin.jpg]] | [[Image:HW2_CTfunction_ECE301Fall2008mboutin.jpg]] | ||
| + | === Non-Periodic Discrete Time Signal === | ||
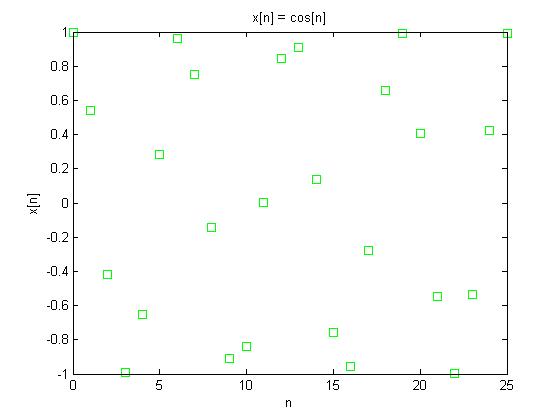
| − | === Periodic | + | Using the CT signal <math> x(t) = cos(t) </math> and converting it to the DT signal <math> x[n] = cos[n] </math> will create a non-periodic function when n is sampled at every integer. |
| + | |||
| + | [[Image:Untitled3_ECE301Fall2008mboutin.jpg]] | ||
| + | |||
| + | === Periodic Discrete Time Signal === | ||
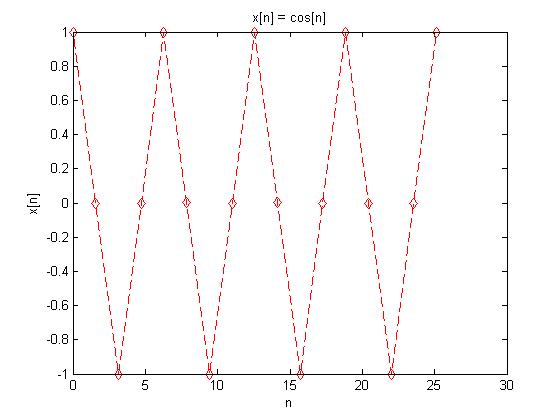
In order to create a discrete time signal <math> x[n] = cos[n] </math> that was still periodic, the time interval couldn't be integers, as shown previously. Therefore, a time interval of <math> \pi/2 </math> was selected. | In order to create a discrete time signal <math> x[n] = cos[n] </math> that was still periodic, the time interval couldn't be integers, as shown previously. Therefore, a time interval of <math> \pi/2 </math> was selected. | ||
[[Image:Untitled2_ECE301Fall2008mboutin.jpg]] | [[Image:Untitled2_ECE301Fall2008mboutin.jpg]] | ||
| + | |||
| + | |||
| + | |||
| + | === Creating a Periodic from a Non-Periodic Function === | ||
| + | |||
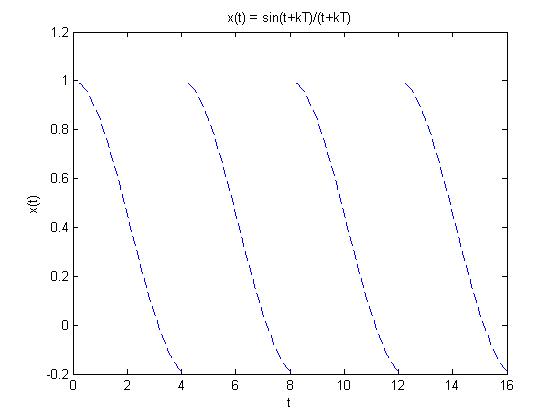
| + | Using <math> x(t) = \frac{sin(t)}{t} </math>, which is a non-periodic function, we can create a periodic function by repeating the original function <math> x(t) </math> from <math> t = [0,4] </math> an infinite number of times. | ||
| + | |||
| + | |||
| + | [[Image:Untitled4_ECE301Fall2008mboutin.jpg]] | ||
Latest revision as of 10:07, 10 September 2008
Contents
Part A: Periodic Signals Revisited
Periodic Continuous Time Signal
I used the continuous time signal $ x(t) = cos(t) $, as it seemed many people used in Homework 1 for their example of a periodic function. The signal repeats itself at intervals of $ 2\pi $.
Non-Periodic Discrete Time Signal
Using the CT signal $ x(t) = cos(t) $ and converting it to the DT signal $ x[n] = cos[n] $ will create a non-periodic function when n is sampled at every integer.
Periodic Discrete Time Signal
In order to create a discrete time signal $ x[n] = cos[n] $ that was still periodic, the time interval couldn't be integers, as shown previously. Therefore, a time interval of $ \pi/2 $ was selected.
Creating a Periodic from a Non-Periodic Function
Using $ x(t) = \frac{sin(t)}{t} $, which is a non-periodic function, we can create a periodic function by repeating the original function $ x(t) $ from $ t = [0,4] $ an infinite number of times.