(New page: Given the sawtooth wave function <math>\,x(t)\,</math>: Image:Jkubasci Sawtooth.jpg == Energy == Compute the energy from 0 to <math>2\pi</math>: == Power == Computer the power from...) |
(→Power) |
||
| (2 intermediate revisions by the same user not shown) | |||
| Line 4: | Line 4: | ||
== Energy == | == Energy == | ||
| − | Compute the energy from 0 to <math>2\pi</math>: | + | Compute the energy from 0 to <math>2\pi</math>. |
| + | |||
| + | Since we're integrating from 0 to <math>2\pi</math>, we can treat this as a simple line <math>\,y(t)=\frac{1}{\pi}t\,</math>. | ||
| + | |||
| + | Answer: <math>\,\frac{8\pi}{3}\,</math> | ||
== Power == | == Power == | ||
| − | Computer the power from 0 to <math>2\pi</math>: | + | Computer the power from 0 to <math>2\pi</math>. |
| + | |||
| + | Answer: <math>\,\frac{4}{3}\,</math> | ||
Latest revision as of 20:30, 4 September 2008
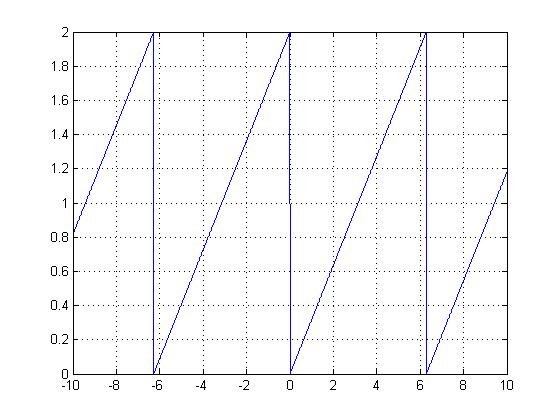
Given the sawtooth wave function $ \,x(t)\, $:
Energy
Compute the energy from 0 to $ 2\pi $.
Since we're integrating from 0 to $ 2\pi $, we can treat this as a simple line $ \,y(t)=\frac{1}{\pi}t\, $.
Answer: $ \,\frac{8\pi}{3}\, $
Power
Computer the power from 0 to $ 2\pi $.
Answer: $ \,\frac{4}{3}\, $