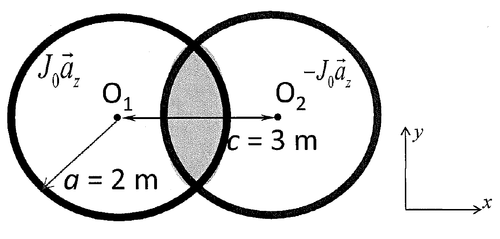
Line 32: Line 32:
  
 
=Solution=
 
=Solution=
−
[[Image:cube.png|Alt text|300x300px]]
+
:'''Click [[ECE_PhD_QE_FO_2013_Problem2.1|here]] to view student [[ECE_PhD_QE_FO_2013_Problem2.1|answers and discussions]]'''
−
 
+
−
<math>
+
−
\begin{align*}
+
−
\nabla \cdot \bar{D} &= \rho \\
+
−
\quad (\frac{\partial}{\partial x}\hat{x}+\frac{\partial}{\partial y}\hat{y}+\frac{\partial}{\partial z}\hat{z})\cdot(2\hat{x})&=\rho \\
+
−
\frac{\partial}{\partial x}(2)&=0=\rho \quad \text{(no charge)}
+
−
\end{align*}
+
−
</math>
+
−
 
+
−
Also:
+
−
 
+
−
<math>
+
−
\begin{align*}
+
−
\oint \bar{D}\cdot d\bar{S}&=Q\\
+
−
&=\int2(dS_x)+\int2(-dS_x)=2-2=\boxed{0}
+
−
\end{align*}
+
−
</math>
+
  
 
==Question 3==
 
==Question 3==
Line 57: Line 40:
  
 
=Solution=
 
=Solution=
−
Using superposition <br/>
+
:'''Click [[ECE_PhD_QE_FO_2013_Problem3.1|here]] to view student [[ECE_PhD_QE_FO_2013_Problem3.1|answers and discussions]]'''
−
In the left cylinder <br/>
+
−
<math>
+
−
\begin{equation*}
+
−
\nabla\times \bar{H}=\bar{J} \longrightarrow \oint \bar{H}\cdot d\bar{l}=\int_S\bar{J}\cdot d\bar{S}
+
−
\qquad \left\{ \begin{aligned}
+
−
dl&=dr\hat{r}+rd\phi\hat{\phi}+dz\hat{z}\\
+
−
d\bar{S}_z&=rd\phi dr\hat{z}
+
−
\end{aligned} \right.
+
−
\end{equation*}
+
−
</math>
+
−
<br/>
+
−
<math>
+
−
\begin{align*}
+
−
\int_0^{2\pi}H_{\phi}(rd\phi)&=\int_0^r\int_0^{2\pi} J_0(r'd\phi dr')\\
+
−
H_{\phi}(2\pi r)&=2\pi J_0(\frac{r^2}{2}) \\
+
−
& \boxed{\bar{H}=\frac{J_0r}{2}\hat{\phi}}
+
−
\end{align*}
+
−
</math>
+
−
<br/>
+
−
[[Image:cil.png|Alt text|300x300px]]
+
−
<br/>
+
−
<math>
+
−
\begin{align*}
+
−
\text{Transform to cartesian:}&\left\{\begin{aligned}
+
−
r&=\sqrt{x^2+y^2}\\
+
−
\hat{\phi}&=-sin\phi\hat{x}+cos\phi\hat{y}\\
+
−
&=(\frac{-y}{\sqrt{x^2+y^2}})\hat{x}+(\frac{x}{\sqrt{x^2+y^2}})\hat{y}
+
−
\end{aligned}\right. \\
+
−
& \boxed{\bar{H}_L=\frac{J_0}{2}\left[-y\hat{x}+x\hat{y}\right]}
+
−
\end{align*}
+
−
</math>
+
−
 
+
−
In the right cilinder <br/>
+
−
<math>
+
−
\begin{align*}
+
−
&\bar{H}_R=\frac{-J_0}{2}\left[-y'\hat{x'}+x'\hat{y'}\right]&
+
−
\left\{
+
−
\begin{aligned}
+
−
x'&=x-3\\
+
−
y'&=y\\
+
−
\hat{x}'&=\hat{x}\\
+
−
\hat{y}'&=\hat{y}
+
−
\end{aligned}
+
−
\right.\\
+
−
&\boxed{\bar{H}_R=\frac{-J_0}{2}\left[-y\hat{x}+(x-3)\hat{y}\right]}&
+
−
\end{align*}
+
−
</math>
+
−
 
+
−
<math>
+
−
\begin{equation*}
+
−
\boxed{\bar{H}_T=\bar{H}_L+\bar{H}_R=\frac{3J_0}{2}\hat{y}}
+
−
\end{equation*}
+
−
</math>
+
  
 
----
 
----
 
[[ECE_PhD_Qualifying_Exams|Back to ECE Qualifying Exams (QE) page]]
 
[[ECE_PhD_Qualifying_Exams|Back to ECE Qualifying Exams (QE) page]]

Latest revision as of 20:52, 24 April 2017


ECE Ph.D. Qualifying Exam

Fields and Optics (FO)

Question 1: Statics 1

August 2013

Question 1

Alt text

Alt text

Solution

Click here to view student answers and discussions

Question 2

Alt text

Solution

Click here to view student answers and discussions

Question 3

Alt text

Alt text

Solution

Click here to view student answers and discussions

Back to ECE Qualifying Exams (QE) page

Retrieved from "https://www.projectrhea.org/rhea/index.php?title=ECE_PhD_QE_Fields_Optics_2013&oldid=72880"
Shortcuts
Help Main Wiki Page Random Page Special Pages Log in
Search

Alumni Liaison

To all math majors: "Mathematics is a wonderfully rich subject."

Dr. Paul Garrett