| Line 18: | Line 18: | ||
---- | ---- | ||
==Question== | ==Question== | ||
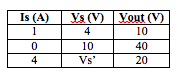
| − | The box represents a linear network containing no independent sources. Given the data in the table below determine the voltage Vs' when Is = | + | The box represents a linear network containing no independent sources. Given the data in the table below determine the voltage Vs' when Is = 4A and Vout = 20V. |
| − | [[File:ECE201_P4_1.jpeg| | + | [[File:ECE201_P4_1.jpeg|250px|center]] |
| − | [[File:ECE201_P4_2.png| | + | [[File:ECE201_P4_2.png|250px|center]] |
---- | ---- | ||
Latest revision as of 14:33, 2 May 2015
Linear Network Practice
Practice question for ECE201: "Linear circuit analysis I"
By: Chinar Dhamija
Topic: Linear Networks
Question
The box represents a linear network containing no independent sources. Given the data in the table below determine the voltage Vs' when Is = 4A and Vout = 20V.
Answer
In order to find Vs' we have the following equation:
$ 20 = Vs'a + 4b $
From the information present in the table two systems of equations can be created to solve for a and b.
First equation:
$ 10 = 4a + b $
Second equation:
$ 40 = 10a + 0b $
After solving the above system of equations you get:
$ a = 4 $ and $ b = -6 $
Since we have both a and b values you can plug that into the Vs' equation and solve for Vs'.
$ \begin{align} 20 = Vs'a + 4b\\ 20 = Vs'(4) + 4(-6)\\ 44 = 4Vs'\\ Vs' = 11 V \end{align} $
Questions and comments
If you have any questions, comments, etc. please post them below
- Comment 1
- Answer to Comment 1
- Comment 2
- Answer to Comment 2