(New page: Image:weee.jpg) |
|||
| (4 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
[[Image:weee_ECE302Fall2008sanghavi.jpg]] | [[Image:weee_ECE302Fall2008sanghavi.jpg]] | ||
| + | |||
| + | Blue line => <math> L/2 = \sqrt{R^2-D^2}</math> | ||
| + | |||
| + | Length <math> = 2*(L/2)=2*\sqrt{R^2-D^2}</math> | ||
| + | |||
| + | let X be the length of chord | ||
| + | |||
| + | |||
| + | <math>X=2*\sqrt{R^2-D^2}</math> if <math> 0<D<R \,\ </math> | ||
| + | |||
| + | <math>X=2*R \,\ </math> if <math>D=0 \,\ </math> | ||
| + | |||
| + | <math>X=0 \,\ </math> | ||
| + | else | ||
| + | |||
| + | so its PDF will be | ||
| + | <math>\int_0^{2*R} \sqrt{R^2-D^2} dD</math> | ||
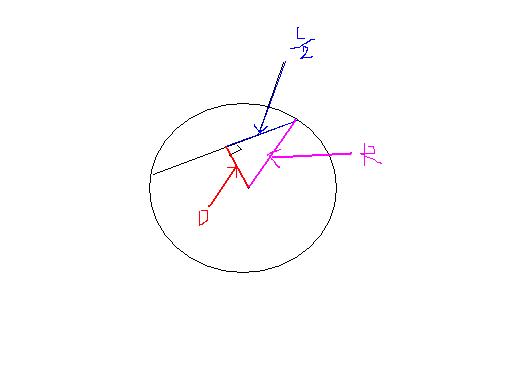
Latest revision as of 09:43, 7 October 2008
Blue line => $ L/2 = \sqrt{R^2-D^2} $
Length $ = 2*(L/2)=2*\sqrt{R^2-D^2} $
let X be the length of chord
$ X=2*\sqrt{R^2-D^2} $ if $ 0<D<R \,\ $
$ X=2*R \,\ $ if $ D=0 \,\ $
$ X=0 \,\ $ else
so its PDF will be $ \int_0^{2*R} \sqrt{R^2-D^2} dD $