| (19 intermediate revisions by the same user not shown) | |||
| Line 5: | Line 5: | ||
[[Category:image processing]] | [[Category:image processing]] | ||
| − | = [[ECE_PhD_Qualifying_Exams|ECE Ph.D. Qualifying Exam]] | + | <br> |
| − | + | <center> | |
| + | <font size="4"> [[ECE_PhD_Qualifying_Exams|ECE Ph.D. Qualifying Exam]] </font> | ||
| − | + | <font size="4"> Communication Networks Signal and Image processing (CS) </font> | |
| + | |||
| + | <font size="4"> [[QE637_sol2012|Question 5, August 2012(Published on May 2017)]]</font> | ||
| + | </center> | ||
| + | |||
| + | <font size="4">[[ QE637_sol2012_Q1 | Problem 1]],[[ QE637_sol2012_Q2 |2]]</font> | ||
---- | ---- | ||
| + | |||
== Solution1: == | == Solution1: == | ||
| − | a) | + | a) |
| − | + | ||
| + | The gamma of the device is equal 1. | ||
b) | b) | ||
| Line 39: | Line 47: | ||
\end{align} | \end{align} | ||
</math> | </math> | ||
| − | |||
<math> | <math> | ||
| Line 63: | Line 70: | ||
\end{align} | \end{align} | ||
</math> | </math> | ||
| − | |||
<math> | <math> | ||
| Line 119: | Line 125: | ||
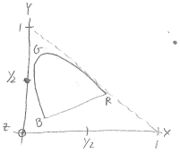
As can be seen, since the point lies outside the horseshoe shape diagram, it’s doesn’t exist (imaginary color) while mathematically we can talk about it and write down the equation for it. For this point, R<0, G>0, and B>0. | As can be seen, since the point lies outside the horseshoe shape diagram, it’s doesn’t exist (imaginary color) while mathematically we can talk about it and write down the equation for it. For this point, R<0, G>0, and B>0. | ||
| − | e) We see false contours in dark region, because small changes in quantization level leads to large contrast changes that cause visible contours. | + | e) |
| + | |||
| + | We see false contours in dark region, because small changes in quantization level leads to large contrast changes that cause visible contours. | ||
== Solution 2: == | == Solution 2: == | ||
| − | a) <math>\left[ {\begin{array}{*{20}{c}} | + | a) |
| + | |||
| + | <math>\left[ {\begin{array}{*{20}{c}} | ||
R\\ | R\\ | ||
G\\ | G\\ | ||
| Line 166: | Line 176: | ||
\end{array}} \right] \Rightarrow (x_b,y_b)=(\frac{c}{c+f+i},\frac{f}{c+f+i}) | \end{array}} \right] \Rightarrow (x_b,y_b)=(\frac{c}{c+f+i},\frac{f}{c+f+i}) | ||
</math> <br \> | </math> <br \> | ||
| − | |||
c) | c) | ||
| Line 179: | Line 188: | ||
1\\ | 1\\ | ||
1\\ | 1\\ | ||
| − | \end{array}} \right] \Rightarrow {{x}_{w}}=\frac{a+b+c}{a+b+c+d+e+f+g+h+i}, {{Y}_{w}}=\frac{d+e+f}{a+b+c+d+e+f+g+h+i} , {{Z}_{w}}=1-{{ | + | \end{array}} \right] \Rightarrow {{x}_{w}}=\frac{a+b+c}{a+b+c+d+e+f+g+h+i}, {{Y}_{w}}=\frac{d+e+f}{a+b+c+d+e+f+g+h+i} , {{Z}_{w}}=1-{{X}_{w}}-{{Y}_{w}}. |
</math> <br \> | </math> <br \> | ||
| + | <span style="color:green"> We use small letters for chromaticity coordinates, so the student should use <math>{{y}_{w}}</math> and <math>{{z}_{w}}</math> instead of <math>{{Y}_{w}}</math> and <math>{{Z}_{w}}.</math></span> | ||
| − | d) [[File: | + | d) |
| + | |||
| + | [[File:sol2_2012_1d.jpg|thumbnail|center]] | ||
R is negative and B and G are positive. | R is negative and B and G are positive. | ||
| − | |||
| − | e) Artifacts in dark regions of gradient where human contrast sensitivity is higher (more sensitive to quantization error). | + | e) |
| + | |||
| + | Artifacts in dark regions of gradient where human contrast sensitivity is higher (more sensitive to quantization error). | ||
| + | [[File:sol2_2012_1e.jpg|thumbnail|center]] | ||
| + | |||
| + | <span style="color:green"> The student didn't mention what the type of artifacts, which is false contours.</span> | ||
---- | ---- | ||
===Related Problem=== | ===Related Problem=== | ||
| − | Consider | + | Consider the color display that produces the following color (X,Y,Z) when given the input is (r, g, b). |
| − | <math> | + | <center> |
| − | \left[ | + | <math>\left[ \begin{matrix} |
| − | X\\ | + | X \\ |
| − | Y\\ | + | Y \\ |
| − | Z | + | Z \\ |
| − | \end{ | + | \end{matrix} \right]=\left[ \begin{matrix} |
| − | + | 1 & 0 & 0 \\ | |
| − | + | 0 & 1 & 0 \\ | |
| − | + | 0 & 0 & 1 \\ | |
| − | \end{ | + | \end{matrix} \right]\left[ \begin{matrix} |
| − | + | {{(r/255)}^{\alpha }} \\ | |
| − | g^\alpha\\ | + | {{(g/255)}^{\alpha }} \\ |
| − | b^\alpha | + | {{(b/255)}^{\alpha }} \\ |
| − | \end{ | + | \end{matrix} \right]</math> |
| − | </math> | + | </center> |
| + | a) What is the gamma of the display? | ||
| + | |||
| + | b) What is the white point of the display? | ||
| + | |||
| + | c) What are the color primaries of the display? | ||
| + | |||
| + | d) Can such a display be physically built? Why or why not? | ||
| − | a) | + | e) You produce two images, one with a checker board pattern of values 0 and 255, and a second with a constant value of (r, g, b) = (a, a, a). The value of a is then adjusted so that the two images match when viewed from a distance. What is the value of gamma for the display in terms of the value a? |
| − | + | f) Imagine that <math>\alpha =1</math> and the values of (r, g, b) are quantized to 8-bits. Describe the defects you would expect to see in the displayed image. | |
| − | + | Refer to [https://engineering.purdue.edu/~bouman/ece637/previous/ece637S2010/exams/exam2/exam.pdf <u>ECE637 Spring 2010 Exam2 Problem2</u>].<br> | |
| − | |||
---- | ---- | ||
[[ECE_PhD_Qualifying_Exams|Back to ECE QE page]]: | [[ECE_PhD_Qualifying_Exams|Back to ECE QE page]]: | ||
Latest revision as of 18:43, 2 May 2017
Communication Networks Signal and Image processing (CS)
Problem 1,2
Solution1:
a)
The gamma of the device is equal 1.
b)
$ \begin{align} & \left[ \begin{matrix} {{X}_{r}} \\ {{Y}_{r}} \\ {{Z}_{r}} \\ \end{matrix} \right]=\left[ \begin{matrix} a & b & c \\ d & e & f \\ g & h & i \\ \end{matrix} \right]\left[ \begin{matrix} 1 \\ 0 \\ 0 \\ \end{matrix} \right]\Rightarrow \left\{ \begin{matrix} {{X}_{r}}=a \\ {{Y}_{r}}=d \\ {{Z}_{r}}=g \\ \end{matrix} \right. \\ & {{x}_{r}}={{\frac{{{X}_{r}}}{{{X}_{r}}+{{Y}_{r}}+Z}}_{r}}=\frac{a}{a+d+g},_{{}}^{{}}{{y}_{r}}=\frac{{{Y}_{r}}}{{{X}_{r}}+{{Y}_{r}}+{{Z}_{r}}}=\frac{d}{a+d+g} \\ \end{align} $
$ \begin{align} & \left[ \begin{matrix} {{X}_{g}} \\ {{Y}_{g}} \\ {{Z}_{g}} \\ \end{matrix} \right]=\left[ \begin{matrix} a & b & c \\ d & e & f \\ g & h & i \\ \end{matrix} \right]\left[ \begin{matrix} 0 \\ 1 \\ 0 \\ \end{matrix} \right]\Rightarrow \left\{ \begin{matrix} {{X}_{g}}=b \\ {{Y}_{g}}=e \\ {{Z}_{g}}=h \\ \end{matrix} \right. \\ & {{x}_{g}}=\frac{{{X}_{g}}}{{{X}_{g}}+{{Y}_{g}}+{{Z}_{g}}}=\frac{b}{b+e+h},_{{}}^{{}}{{y}_{g}}=\frac{{{Y}_{g}}}{{{X}_{g}}+{{Y}_{g}}+{{Z}_{g}}}=\frac{e}{b+e+h} \\ \end{align} $
$ \begin{align} & \left[ \begin{matrix} {{X}_{b}} \\ {{Y}_{b}} \\ {{Z}_{b}} \\ \end{matrix} \right]=\left[ \begin{matrix} a & b & c \\ d & e & f \\ g & h & i \\ \end{matrix} \right]\left[ \begin{matrix} 0 \\ 0 \\ 1 \\ \end{matrix} \right]\Rightarrow \left\{ \begin{matrix} {{X}_{b}}=c \\ {{Y}_{b}}=f \\ {{Z}_{b}}=i \\ \end{matrix} \right. \\ & {{x}_{b}}=\frac{{{X}_{b}}}{{{X}_{b}}+{{Y}_{b}}+{{Z}_{b}}}=\frac{c}{c+f+i},_{{}}^{{}}{{y}_{b}}=\frac{{{Y}_{b}}}{{{X}_{b}}+{{Y}_{b}}+{{Z}_{b}}}=\frac{f}{c+f+i} \\ \end{align} $
c)
$ \begin{align} & \left[ \begin{matrix} {{X}_{w}} \\ {{Y}_{w}} \\ {{Z}_{w}} \\ \end{matrix} \right]=\left[ \begin{matrix} a & b & c \\ d & e & f \\ g & h & i \\ \end{matrix} \right]\left[ \begin{matrix} 1 \\ 1 \\ 1 \\ \end{matrix} \right]\Rightarrow \left\{ \begin{matrix} {{X}_{w}}=a+b+c \\ {{Y}_{w}}=d+e+f \\ {{Z}_{w}}=g+h+i \\ \end{matrix} \right. \\ & A={{X}_{w}}+{{Y}_{w}}+{{Z}_{w}}=a+b+c+d+e+f+g+h+i \\ & {{x}_{w}}=\frac{{{X}_{w}}}{{{X}_{w}}+{{Y}_{w}}+{{Z}_{w}}}=\frac{a+b+c}{A},_{{}}^{{}}{{y}_{w}}=\frac{{{Y}_{w}}}{{{X}_{w}}+{{Y}_{w}}+{{Z}_{w}}}=\frac{d+e+f}{A} \\ \end{align} $
d)
$ (X,Y,Z)=(0,1/2,1/2)_{{}}^{{}}\Rightarrow _{{}}^{{}}(x,y)=(0,1/2) $As can be seen, since the point lies outside the horseshoe shape diagram, it’s doesn’t exist (imaginary color) while mathematically we can talk about it and write down the equation for it. For this point, R<0, G>0, and B>0.
e)
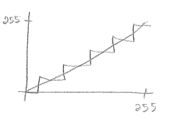
We see false contours in dark region, because small changes in quantization level leads to large contrast changes that cause visible contours.
Solution 2:
a)
$ \left[ {\begin{array}{*{20}{c}} R\\ G\\ B \end{array}} \right] $ are linear with energy $ \Rightarrow $ $ \gamma=1 $.
b)
$ \left[ {\begin{array}{*{20}{c}} X\\ Y\\ Z \end{array}} \right]=M\left[ {\begin{array}{*{20}{c}} 1\\ 0\\ 0\\ \end{array}} \right] \Rightarrow (x_r,y_r)=(\frac{a}{a+d+g},\frac{d}{a+d+g}) $
$ \left[ {\begin{array}{*{20}{c}} X\\ Y\\ Z \end{array}} \right]=M\left[ {\begin{array}{*{20}{c}} 0\\ 1\\ 0\\ \end{array}} \right] \Rightarrow (x_g,y_g)=(\frac{b}{b+e+h},\frac{e}{b+e+h}) $
$ \left[ {\begin{array}{*{20}{c}} X\\ Y\\ Z \end{array}} \right]=M\left[ {\begin{array}{*{20}{c}} 0\\ 0\\ 1\\ \end{array}} \right] \Rightarrow (x_b,y_b)=(\frac{c}{c+f+i},\frac{f}{c+f+i}) $
c)
$ \left[ {\begin{array}{*{20}{c}} X\\ Y\\ Z \end{array}} \right]=M\left[ {\begin{array}{*{20}{c}} 1\\ 1\\ 1\\ \end{array}} \right] \Rightarrow {{x}_{w}}=\frac{a+b+c}{a+b+c+d+e+f+g+h+i}, {{Y}_{w}}=\frac{d+e+f}{a+b+c+d+e+f+g+h+i} , {{Z}_{w}}=1-{{X}_{w}}-{{Y}_{w}}. $
We use small letters for chromaticity coordinates, so the student should use $ {{y}_{w}} $ and $ {{z}_{w}} $ instead of $ {{Y}_{w}} $ and $ {{Z}_{w}}. $
d)
R is negative and B and G are positive.
e)
Artifacts in dark regions of gradient where human contrast sensitivity is higher (more sensitive to quantization error).
The student didn't mention what the type of artifacts, which is false contours.
Related Problem
Consider the color display that produces the following color (X,Y,Z) when given the input is (r, g, b).
$ \left[ \begin{matrix} X \\ Y \\ Z \\ \end{matrix} \right]=\left[ \begin{matrix} 1 & 0 & 0 \\ 0 & 1 & 0 \\ 0 & 0 & 1 \\ \end{matrix} \right]\left[ \begin{matrix} {{(r/255)}^{\alpha }} \\ {{(g/255)}^{\alpha }} \\ {{(b/255)}^{\alpha }} \\ \end{matrix} \right] $
a) What is the gamma of the display?
b) What is the white point of the display?
c) What are the color primaries of the display?
d) Can such a display be physically built? Why or why not?
e) You produce two images, one with a checker board pattern of values 0 and 255, and a second with a constant value of (r, g, b) = (a, a, a). The value of a is then adjusted so that the two images match when viewed from a distance. What is the value of gamma for the display in terms of the value a?
f) Imagine that $ \alpha =1 $ and the values of (r, g, b) are quantized to 8-bits. Describe the defects you would expect to see in the displayed image.
Refer to ECE637 Spring 2010 Exam2 Problem2.