| Line 26: | Line 26: | ||
==Introduction== | ==Introduction== | ||
| − | + | This slecture covers definition of downsampling and demonstrates how to obtain downsampled signal in frequency domain. | |
---- | ---- | ||
Revision as of 10:18, 9 October 2014
Frequency domain view of the relationship between a signal and a sampling of that signal
A slecture by ECE student Yerkebulan Yeshmukhanbetov
Partly based on the ECE438 Fall 2014 lecture material of Prof. Mireille Boutin.
Outline
- Introduction
- Derivation
- Example
- Conclusion
Introduction
This slecture covers definition of downsampling and demonstrates how to obtain downsampled signal in frequency domain.
Derivation
signal: $ x_s(t) $ and $ x_d[n] $. $ x_s(t) $ $ P_T(t) $ with the original signal $ x(t) $ and actually $ x_s(t) $ is $ comb_T(x(t)) $ where T is the sampling period.
However the $ x_d[n] $ is $ x(nT) $ where T is the sampling period.
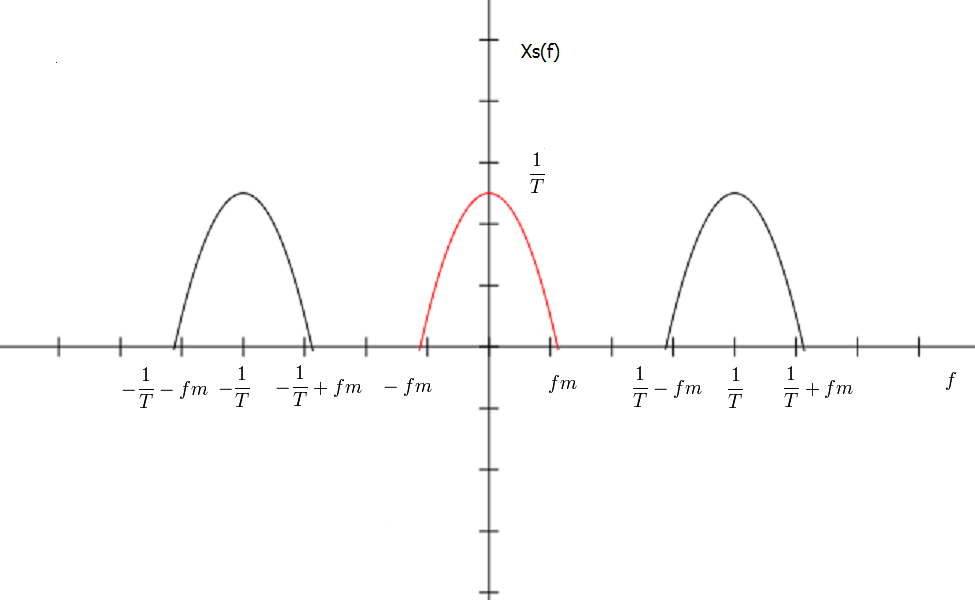
relationship between $ X(f) $ and $ X_s(f) $.
We know that $ x_s(t) = x(t) \times P_T(t) $, we can derive the relationship between $ x_s(t) $ and $ x(t) $ in the following way:
$ \begin{align} F(comb_T(x(t)) &= F(x(t) \times P_T(t))\\ &= X(f)*F(P_T(t))\\ &= X(f)*\frac{1}{T}\sum_{n = -\infty}^\infty \delta(f-\frac{n}{T})\\ &= \frac{1}{T}X(f)*P_\frac{1}{T}(f)\\ &= \frac{1}{T}rep_\frac{1}{T}X(f)\\ \end{align} $
Show this relationship in graph below:
example
Derivation
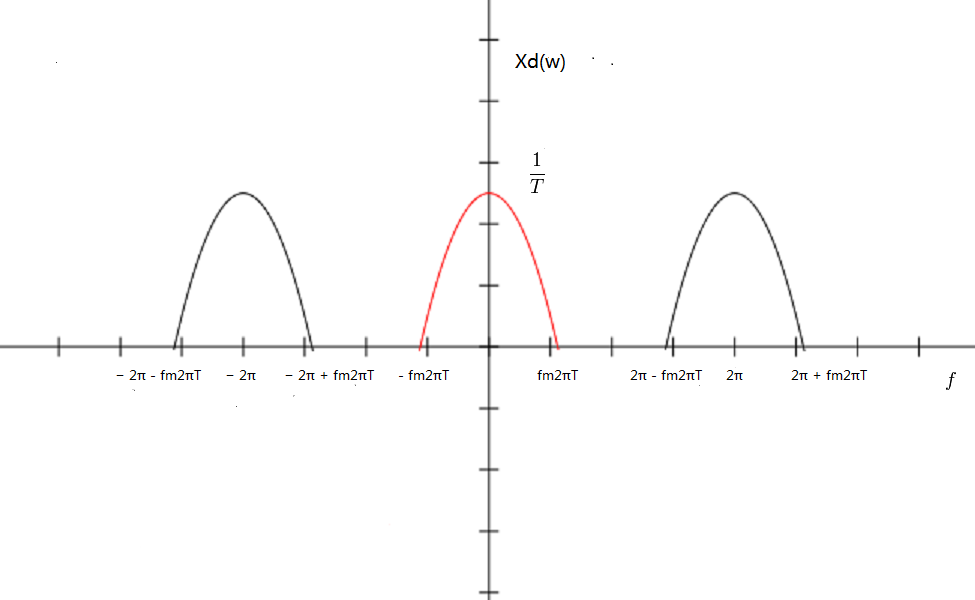
n $ X_s(f) $ and $ X_d(\omega) $
We know another way to express CTFT of $ x_s(t) $:
$ \begin{align} X_s(f) &= F(\sum_{n = -\infty}^\infty x(nT)\delta(t-nT))\\ &= \sum_{n = -\infty}^\infty x(nT)F(\delta(t-nT))\\ &= \sum_{n = -\infty}^\infty x(nT)e^{-j2\pi fnT}\\ \end{align} $
compare it with DTFT of $ x_d[n] $:
$ \begin{align} X_d(\omega) &= \sum_{n = -\infty}^\infty x_d[n]e^{-j\omega n}\\ &= \sum_{n = -\infty}^\infty x(nT)e^{-j\omega n}\\ \end{align} $
we can find that:
$ \begin{align} X_d(2\pi Tf) &= X_s(f)\\ \end{align} $
if $ f = \frac{1}{T} $
we have that:
$ \begin{align} X_d(2\pi ) &= X_s(\frac{1}{T})\\ \end{align} $
ationship between $ X_s(f) $ and $ X_d(\omega) $ and the is showed in graph as below:
example
conclusion
So the relationship between $ X(f) $ and $ X_s(f) $ is that $ X_s(f) $ is a a rep of $ X(f) $ in frequency domain with period of $ \frac{1}{T} $ and magnitude scaled by $ \frac{1}{T} $. the relationship between $ X(f) $ and $ X_d(\omega) $ is that $ X_d(\omega) $ is also a a rep of $ X(f) $ in frequency domain with period $ 2\pi $ and magnitude is also scaled by $ \frac{1}{T} $, but the frequency is scaled by $ 2\pi T $