(→8.8 #54) |
|||
| Line 8: | Line 8: | ||
It does not converge for me either, but Josh, be careful with your comparison. It's not really valid to use <math>1/\sqrt{x^4}</math> as the function for comparison, because <math>x/\sqrt{x^4} < x/\sqrt{x^4}</math>, albeit infinitesimally less. I used <math>(g(x) = 1/x^{0.99}) > (f(x) = x/\sqrt{x^4}) </math>, in which case P < 1 and diverges. --[[User:Reckman|Randy Eckman]] 15:44, 26 October 2008 (UTC) | It does not converge for me either, but Josh, be careful with your comparison. It's not really valid to use <math>1/\sqrt{x^4}</math> as the function for comparison, because <math>x/\sqrt{x^4} < x/\sqrt{x^4}</math>, albeit infinitesimally less. I used <math>(g(x) = 1/x^{0.99}) > (f(x) = x/\sqrt{x^4}) </math>, in which case P < 1 and diverges. --[[User:Reckman|Randy Eckman]] 15:44, 26 October 2008 (UTC) | ||
| + | |||
| + | == Pg. 329, #16 == | ||
| + | |||
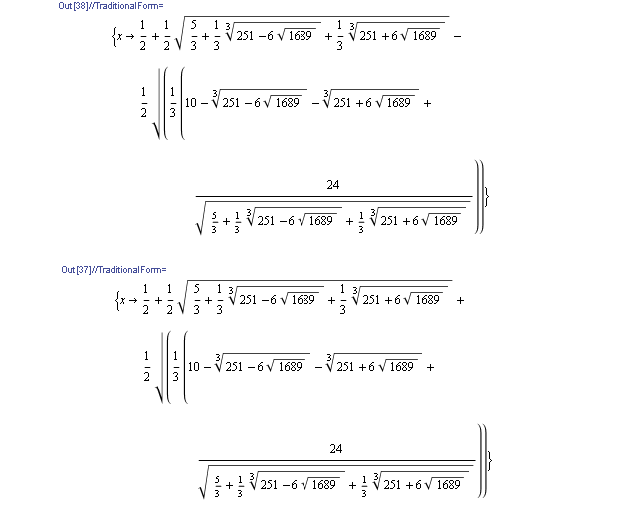
| + | How accurate do we need to make our answers for the roots? After four iterations, I have the first point accurate to four digits. The text doesn't specify a number of correct digits, and out of curiosity I found the precise roots on Mathematica. I don't know how the textbook could expect us to calculate exactly this: | ||
| + | |||
| + | [[Image:Mathematicapg329num16_MA181Fall2008bell.PNG]] | ||
| + | |||
| + | {x -> 0.630115} | ||
| + | {x -> 2.57327} | ||
| + | |||
| + | --[[User:Reckman|Randy Eckman]] 17:43, 26 October 2008 (UTC) | ||
Revision as of 12:43, 26 October 2008
8.8 #54
Does this indefinite integral converge for anyone? Also, if you are having trouble with the integral, take a look at the derivatives of inverse hyperbolic functions. --John Mason
It does not converge for me. I used direct comparison to test whether it converges or not. I started by comparing $ \sqrt{x^4-1} $ and $ \sqrt{x^4} $ It was easy from there. --Josh Visigothsandwich
Thanks. I'm not really sure that I needed to use some sort of comparison to show it didn't converge, as it did integrate nicely, but its good to have a second opinion, be that mathematical or otherwise. --John Mason
It does not converge for me either, but Josh, be careful with your comparison. It's not really valid to use $ 1/\sqrt{x^4} $ as the function for comparison, because $ x/\sqrt{x^4} < x/\sqrt{x^4} $, albeit infinitesimally less. I used $ (g(x) = 1/x^{0.99}) > (f(x) = x/\sqrt{x^4}) $, in which case P < 1 and diverges. --Randy Eckman 15:44, 26 October 2008 (UTC)
Pg. 329, #16
How accurate do we need to make our answers for the roots? After four iterations, I have the first point accurate to four digits. The text doesn't specify a number of correct digits, and out of curiosity I found the precise roots on Mathematica. I don't know how the textbook could expect us to calculate exactly this:
{x -> 0.630115} {x -> 2.57327}
--Randy Eckman 17:43, 26 October 2008 (UTC)