| Line 14: | Line 14: | ||
But we had taken the derivative of z(t) to get g(t) (and hence <math> g_k </math>). | But we had taken the derivative of z(t) to get g(t) (and hence <math> g_k </math>). | ||
<math> \therefore z_k = \left ( \frac{g_k}{jk\omega_o} \right ) </math> | <math> \therefore z_k = \left ( \frac{g_k}{jk\omega_o} \right ) </math> | ||
| + | |||
| + | <math> z_k = \left( \frac {1}{k\pi} \sin ( \frac {k\pi}{2} ) * (1 - (-1)^k) \right) | ||
Revision as of 11:29, 1 July 2008
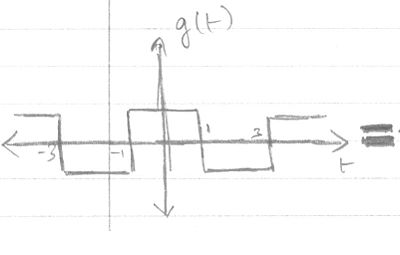
Let $ g(t) = \left ( \frac{dz}{dt} \right ) $
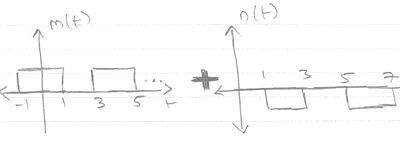
Therefore, $ m_k = \left ( \frac {1}{k\pi} \sin ( \frac {k\pi}{2} ) \right) , n_k = \left( \frac {-1}{k\pi} \sin ( \frac {k\pi}{2} ) e^\frac{-j2k\pi2}{4} \right) $
But $ g_k = m_k + n_k = \left ( \frac {1}{k\pi} \sin ( \frac {k\pi}{2} ) \right) + \left( \frac {-1}{k\pi} \sin ( \frac {k\pi}{2} ) e^\frac{-j2k\pi2}{4} \right) $
$ \therefore g_k = \left ( \frac {1}{k\pi} \sin ( \frac {k\pi}{2} ) \right) + \left( \frac {-1}{k\pi} \sin ( \frac {k\pi}{2} ) (-1)^k \right) $
But we had taken the derivative of z(t) to get g(t) (and hence $ g_k $). $ \therefore z_k = \left ( \frac{g_k}{jk\omega_o} \right ) $
$ z_k = \left( \frac {1}{k\pi} \sin ( \frac {k\pi}{2} ) * (1 - (-1)^k) \right) $