(→AM Demodulation) |
|||
| Line 1: | Line 1: | ||
== AM Demodulation == | == AM Demodulation == | ||
| − | <math>r(n)= x(n) | + | <math>r(n)= x(n)cos^2(n \theta)= \frac{1}{2} x(n) + \frac{1}{2}x(n)cos(2n\theta)</math><br> |
| − | <math> | + | <math>Y(e^{j\theta})= R(e^{j\theta})H(e^{j\theta})</math><br> |
| − | + X(e^{j(\theta)</math> | + | <math> = \frac{1}{2}X(e^{j\theta})+\frac{1}{4}X(e^{j\theta-2\phi})+\frac{1}{4}X(e^{j\theta+2\phi})H(e^{j\theta})</math><br> |
| + | <math> = X(e^{j\theta}) </math> | ||
[[Image:Hw9_ECE301Fall2008mboutin.JPG]] | [[Image:Hw9_ECE301Fall2008mboutin.JPG]] | ||
Revision as of 16:33, 17 November 2008
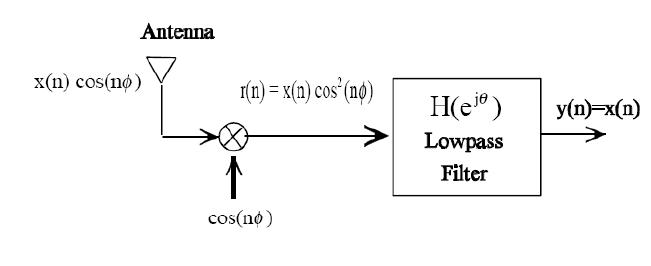
AM Demodulation
$ r(n)= x(n)cos^2(n \theta)= \frac{1}{2} x(n) + \frac{1}{2}x(n)cos(2n\theta) $
$ Y(e^{j\theta})= R(e^{j\theta})H(e^{j\theta}) $
$ = \frac{1}{2}X(e^{j\theta})+\frac{1}{4}X(e^{j\theta-2\phi})+\frac{1}{4}X(e^{j\theta+2\phi})H(e^{j\theta}) $
$ = X(e^{j\theta}) $