| Line 33: | Line 33: | ||
<math>a_{1} = \frac{1}{4} \sum_{n=0}^{3} x[n] e^{-j \frac{\pi}{2} n} = \frac{1}{4}[(0)(1)+(1)(0)+(2)(-1)+(1)(0)]</math> | <math>a_{1} = \frac{1}{4} \sum_{n=0}^{3} x[n] e^{-j \frac{\pi}{2} n} = \frac{1}{4}[(0)(1)+(1)(0)+(2)(-1)+(1)(0)]</math> | ||
| − | |||
'''<math>a_{1} = -\frac{1}{2}</math>''' | '''<math>a_{1} = -\frac{1}{2}</math>''' | ||
| − | |||
Revision as of 17:00, 23 September 2008
Periodic DT Signal
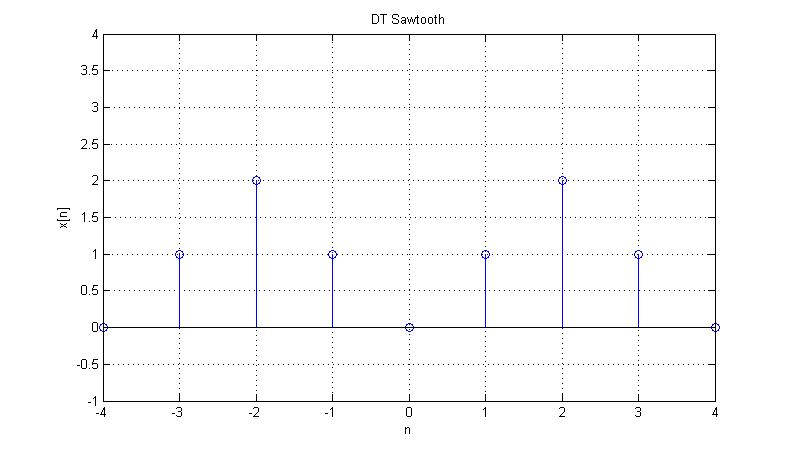
The following plot shows two periods of the periodic DT signal $ x[n] $, a sawtooth:
Fourier Series Coefficients
$ a_{k} = \frac{1}{N} \sum_{n=0}^{N-1} x[n] e^{-jk \frac{2 \pi}{N} n} $
From the plot above, N = 4:
$ a_{k} = \frac{1}{4} \sum_{n=0}^{3} x[n] e^{-jk \frac{\pi}{2} n} $
and:
$ x[0] = 0 $
$ x[1] = 1 $
$ x[2] = 2 $
$ x[3] = 1 $
$ x[4] = 0 $
Therefore:
$ a_{0} = \frac{1}{4} \sum_{n=0}^{3} x[n] = \frac{1}{4}(0+1+2+1) $
$ a_{0} = 1 $
$ a_{1} = \frac{1}{4} \sum_{n=0}^{3} x[n] e^{-j \frac{\pi}{2} n} = \frac{1}{4}[(0)(1)+(1)(0)+(2)(-1)+(1)(0)] $
$ a_{1} = -\frac{1}{2} $