(→Basics of Linearity) |
(→Basics of Linearity) |
||
| Line 10: | Line 10: | ||
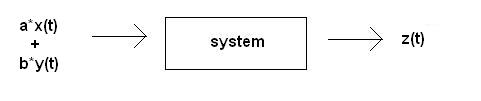
* The system is Linear. | * The system is Linear. | ||
| + | |||
| + | |||
| + | We can break down the input <math> \,\ x(t) = cos(2t) </math> into <math> \,\ x(t) = \frac{1}{2} * (e</math><sup>(j2t)</sup> <math> \,\ + e</math><sup>(-j2t)</sup><math> \,\ )</math>. | ||
| + | |||
| + | Now we can use the property of linearity to determine the output. | ||
Revision as of 13:14, 16 September 2008
Basics of Linearity
We are given the following information:
- For input $ x(t) = e $ (2jt) the output $ y(t) = te $(-2jt).
- For input $ x(t) = e $ (-2jt) the output $ y(t) = te $(2jt).
- The system is Linear.
We can break down the input $ \,\ x(t) = cos(2t) $ into $ \,\ x(t) = \frac{1}{2} * (e $(j2t) $ \,\ + e $(-j2t)$ \,\ ) $.
Now we can use the property of linearity to determine the output.