Proving the Sampling Theorem
The sampling can be represented by "Impulse-train Sampling."
$ x_p(t) = ? $ $ x_p(t) = x(t)p(t) $ $ x_p(t) = x(t)\sum_{n=-\infty}^{\infty} \delta(t-nT) $
We can recover $ x(t) $ from $ x_p(t) $ as follows:
$ x_p(t) \rightarrow H(\omega) \rightarrow x_r(t) $
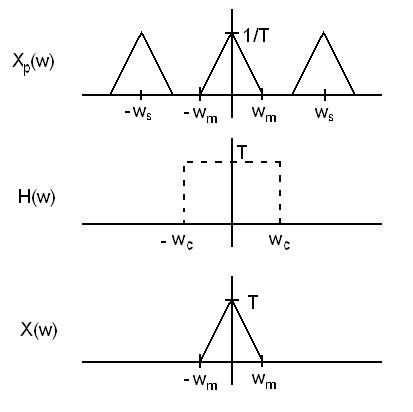
Where $ H(\omega) $ is a filter with gain equal to the period of the signal and a cutoff frequency of $ \omega_c $.
$ \omega_c \rightarrow \omega_m < \omega_c < \omega_s - \omega_m $.
This process can be easily shown in the frequency domain graphically. An example is below.