3)
a)
$ \begin{align*} \text{BC's:}& &E_{1t}&= E_{2t}\\ & &D_{1n}-D_{2n}&=\rho_s \end{align*} $
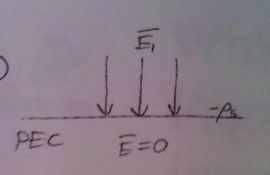
$ \begin{equation*} \text{In PEC, } \bar{E}=0 \longrightarrow \left\{\begin{aligned} E_{1t}&=E_{2t}=0 \\ D_{1n}&=\rho_s \longleftarrow \text{only normal component left equal \\ to charge on surface of PEC} \end{aligned} \right. \end{equation*} $
b)
$ \begin{align*} \bar{E}&=-\nabla V&&\\ &=-\left(\frac{\partial V}{\partial x}\hat{x}+\frac{\partial V}{\partial y}\hat{y}+\frac{\partial V}{\partial z}\hat{z}\right)\\ \end{align*} $
Assume equal potential surface along $ z $ ($ V_{oz} $) then
$ \begin{equation*} \bar{E}=-\left(\frac{\partial V}{\partial x}\hat{x}+\frac{\partial V}{\partial y}\hat{y}\right) \longleftarrow \text{perpendicular to <math>V_{oz}<math>} \end{equation*} $
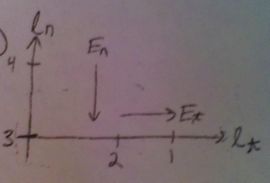
$ \begin{align*} \text{\underline{Also}:}& &V_1-V_2&=-\int_1^2\bar{E}\cdot d\bar{l}&&\\ & &V_0-V_0&=-\int_1^2\bar{E}_t\cdot d\bar{l}_t=0&&\\ & & &\boxed{E_{t}=0}&&\\ & &V_3-V_4&=-\int_3^4\bar{E}\cdot d\bar{l}&&\\ & &V_0-V_4&=-\int_3^4\bar{E}_n\cdot d\bar{l}_n\neq0&&\\ & & &\boxed{E_{n}\neq0}&& \end{align*} $
$ \therefore $ $ \bar{E} $ must $ \bot $ to equal potential surface.